Research on the scale effects of solute transport in a bended karst conduit
-
摘要:
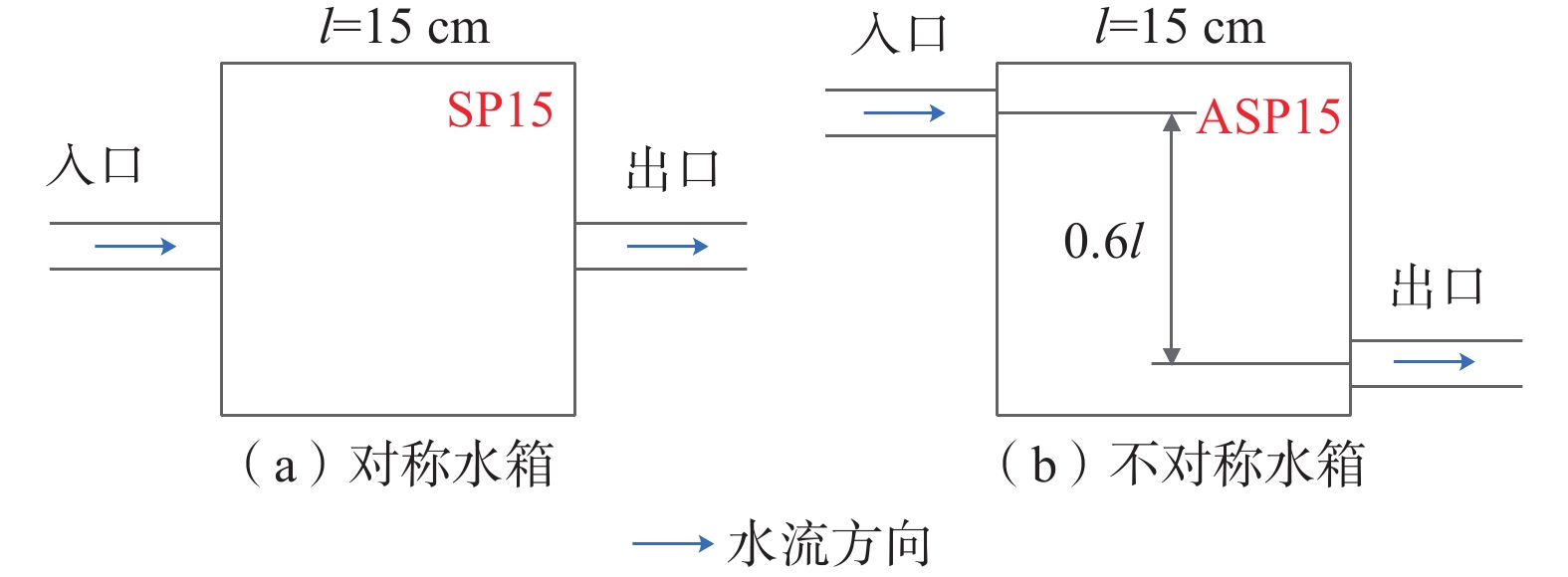
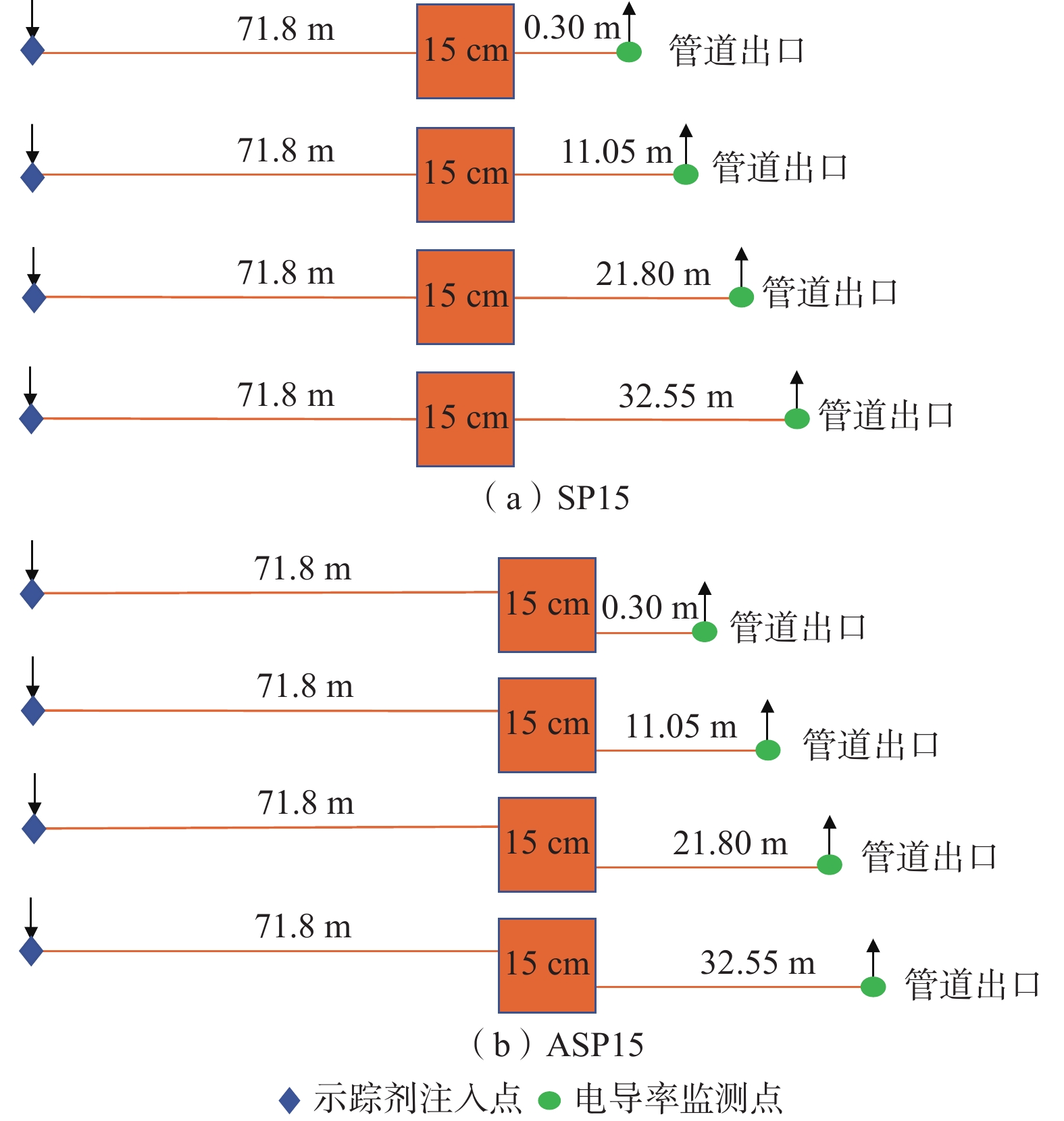
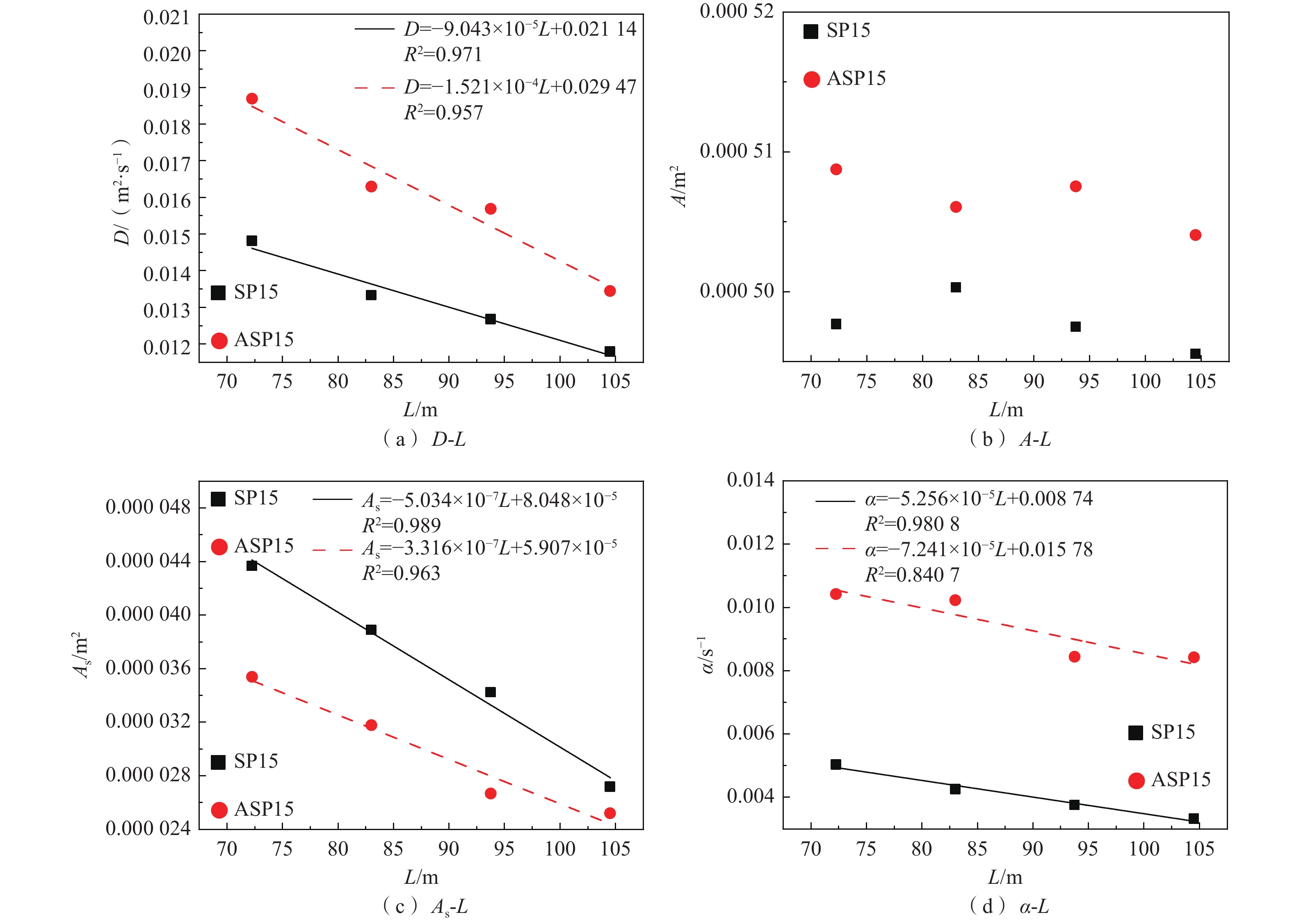
岩溶管道溶质运移的尺度效应研究对穿透曲线的正确解译非常重要,但目前针对单一弯曲管道中溶质运移尺度效应的研究仍比较缺乏。文章将岩溶管道和溶潭分别概化为透明软管和水箱,基于前期建立的水箱-管道系统(简称“管道系统”),在水箱下游设置不同长度的弯曲管道,通过示踪试验研究管道运移尺度对穿透曲线的影响,并采用暂时存储模型模拟试验曲线。结果表明:(1)随着水箱下游管道长度的增加,峰值质量浓度逐渐缓慢降低,穿透曲线上升段斜率无明显变化,穿透曲线拖尾逐渐缩短,表明运移管道长度增加对溶质运移的影响大于下游管道弯曲;(2)穿透曲线偏度系数、后段溶质羽穿透时间和溶质羽穿透时间与管道系统长度呈良好的负线性相关关系(R2>0.96);(3)当对称和不对称水箱管道系统长度分别增加至154.5 m和164.3 m时,偏度系数接近0,穿透曲线分布接近对称;(4)弥散系数、存储区截面积和交换系数与管道系统长度呈良好的负线性相关关系,当对称和不对称水箱管道系统长度分别增加至159.9 m和178.1 m时,存储区截面积接近0,水箱导致的溶质运移滞后效应基本消失。研究结果对野外岩溶管道穿透曲线的解译具有一定指示作用。
Abstract:Research on the scale effect of solute transport in karst conduits is very important for the correct interpretation of breakthrough curves (BTCs), but the scale effect of solute transport in a bended conduit has not been examined. In this paper, a karst conduit and a pool developed within the conduit are generalized into the transparent hose and cubic pool, respectively. Based on the previously established pool-pipe system, the bended pipes with different lengths are arranged downstream of the pool, and the tracer experiments are conducted to study the effect of the transport scale on the BTCs in conduits. We use the transient storage model to simulate the experimental curves. The results show that with the increasing pipe length downstream the pool, the peak concentration gradually decreases slowly, the rising slope of the BTCs does not change significantly, and the BTC tails gradually shorten, indicating that the longer transport distance exerts a larger effect on solute transport than the conduit bend. The coefficient of skewness (CSK), breakthrough time of posterior solute plume (tre) and breakthrough time of solute plume (td) are well negatively correlated with the length of the pipe system (R2>0.96). When the length of the pipe system with the symmetrical or asymmetrical pool increases to 154.5 m and 164.3 m, respectively, the CSK is close to zero, and the BTC is nearly symmetrical. The dispersion coefficient (D), cross-sectional area of the storage zones (As) and exchange coefficient (α) have a good negative correlation with the length of the pipe system. When the length of the pipe system with the symmetrical or asymmetrical pool increases to 159.9 m and 178.1 m, respectively, the As is close to zero. Then, the solute retention caused by the pool basically disappears. The results have certain indications for the interpretation of the BTCs in field karst conduits.
-
Key words:
- karst conduit /
- bend /
- solute transport /
- scale effect /
- tracer experiment
-

-
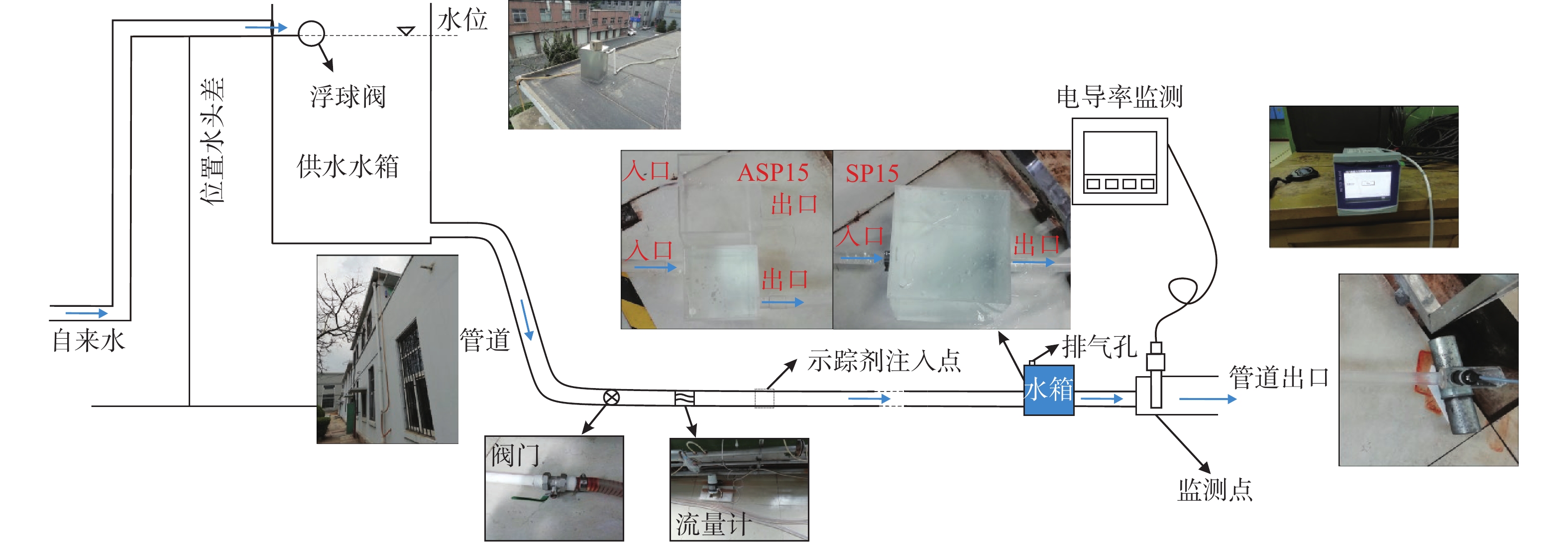
图 1 试验装置示意图(修改自文献[17])
Figure 1.
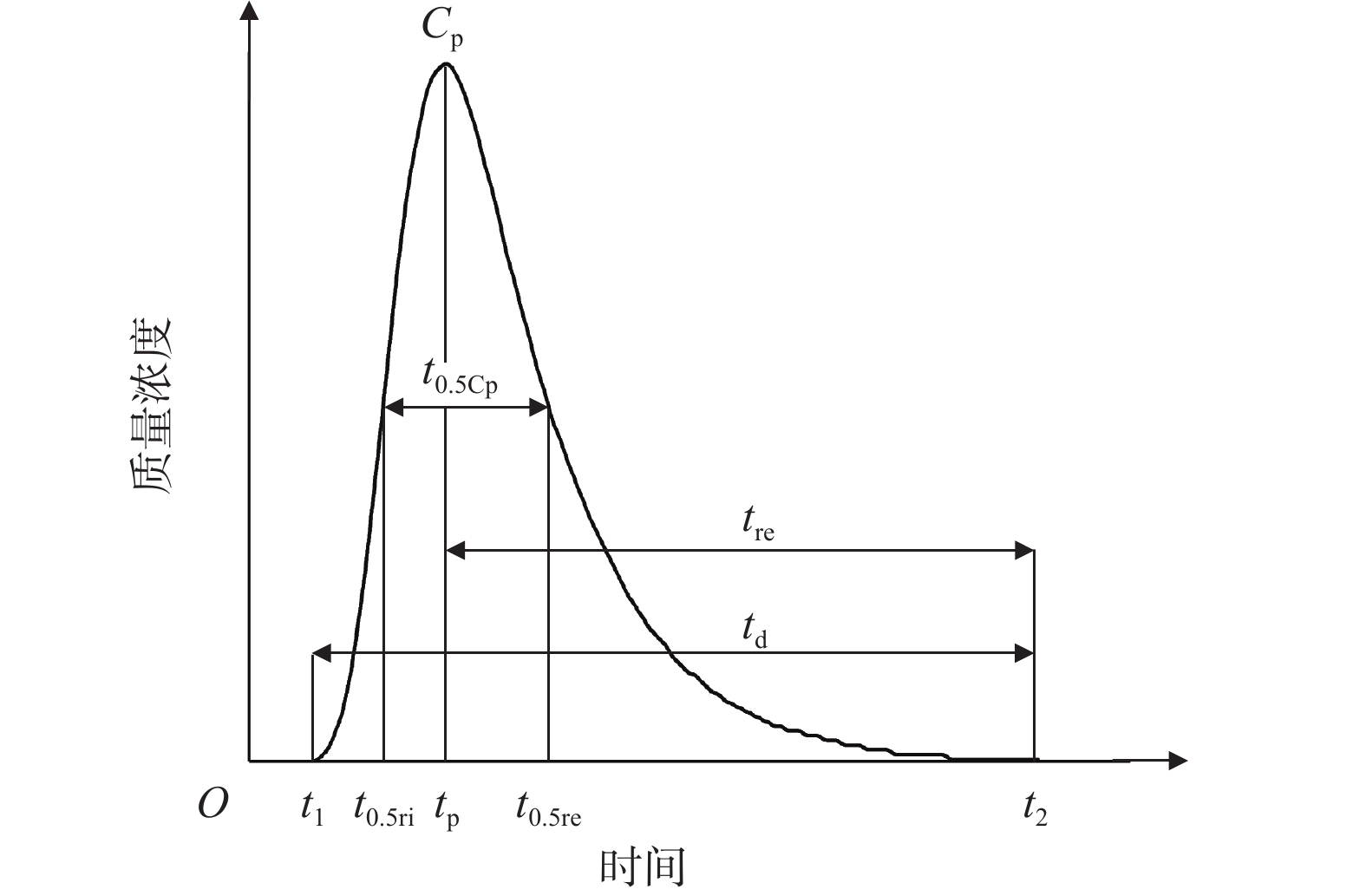
表 1 穿透曲线特征参数
Table 1. Characteristic parameters of breakthrough curve
特征参数 参数分类 计算方法 Cp 最大示踪剂浓度 
Rt 示踪剂回收率 
tm 水力参数 
v 水力参数 
σt2 水力参数 
CSK 水力参数 
tre 水力参数 
t0.5Cp 水力参数 
td 水力参数 
注:C(t)为在任意t时刻的质量浓度;Q(t)为t时刻的流量;M为示踪剂注入质量。 表 2 不同管道系统长度的穿透曲线特征参数
Table 2. Characteristic parameters of breakthrough curve of the pipe system with different lengths
水箱结构 L/m v/(m·s−1) tm/min σt2/min2 CSK tre/s t0.5Cp/s td/s Rt/% SP15 72.25 0.447 2.7004 0.15768 0.1472 175 12 187 91.97 83.00 0.450 3.0745 0.15320 0.1274 164 12 178 91.92 93.75 0.458 3.4124 0.14015 0.1097 148 13 161 92.50 104.50 0.467 3.7317 0.10996 0.0889 125 13 137 89.27 ASP15 72.25 0.446 2.7016 0.052305 0.0847 89 20 105 94.99 83.00 0.452 3.0594 0.048710 0.0722 82 21 98 96.88 93.75 0.457 3.4227 0.045040 0.0620 74 21 90 91.83 104.50 0.461 3.7739 0.044096 0.0557 63 21 79 92.36 表 3 不同管道系统长度的模型参数
Table 3. Model parameters of the pipe system with different lengths
水箱结构 L/m D/(m2·s−1) A/m2 As/m2 α/s−1 Vs/m3 DaI SP15 72.25 1.48×10−2 4.98×10−4 4.37×10−5 5.04×10−3 3.16×10−3 13.2 83.00 1.33×10−2 5.00×10−4 3.89×10−5 4.26×10−3 3.23×10−3 12.6 93.75 1.27×10−2 4.97×10−4 3.42×10−5 3.76×10−3 3.21×10−3 12.4 104.50 1.18×10−2 4.96×10−4 2.72×10−5 3.32×10−3 2.84×10−3 13.5 ASP15 72.25 1.87×10−2 5.09×10−4 3.54×10−5 1.04×10−2 2.56×10−3 34.7 83.00 1.63×10−2 5.06×10−4 3.18×10−5 1.02×10−2 2.64×10−3 37.3 93.75 1.57×10−2 5.08×10−4 2.67×10−5 8.44×10−2 2.50×10−3 36.5 104.50 1.34×10−2 5.04×10−4 2.52×10−5 8.42×10−2 2.63×10−3 38.0 -
[1] FORD D C, WILLIAMS P W. Karst hydrogeology and geomorphology[M]. West Sussex, England: John Wiley & Sons Ltd, 2007.
[2] MORALES T,VALDERRAMA I F D,JESÚS A U,et al. Predicting travel times and transport characterization in karst conduits by analyzing tracer-breakthrough curves[J]. Journal of Hydrology,2007,334(1/2):183 − 198.
[3] GOLDSCHEIDER N,MEIMAN J,PRONK M,et al. Tracer tests in karst hydrogeology and speleology[J]. International Journal of speleology,2008,37(1):27 − 40. doi: 10.5038/1827-806X.37.1.3
[4] 杨平恒,袁道先,蓝家程,等. 基于在线高分辨率监测和定量计算的岩溶地下水示踪试验[J]. 西南大学学报(自然科学版),2013,35(2):103 − 108. [YANG Pingheng,YUAN Daoxian,LAN Jiacheng,et al. Tracing test of a karst aquifer based on online,high-resolution monitoring and quantitative calculation[J]. Journal of Southwest University (Natural Science Edition),2013,35(2):103 − 108. (in Chinese with English abstract)
[5] 程亚平,陈余道. 岩溶地下河定量示踪研究方法综述[J]. 桂林理工大学学报,2016,36(2):242 − 246. [CHENG Yaping,CHEN Yudao. Review of quantitative tracing studies on karst underground river[J]. Journal of Guilin University of Technology,2016,36(2):242 − 246. (in Chinese with English abstract)
[6] 赵良杰,杨杨,易连兴,等. 应用物理非平衡CDE模型反演岩溶管道流参数[J]. 工程勘察,2014,42(9):56 − 59. [ZHAO Liangjie,YANG Yang,YI Lianxing,et al. Application of physical nonequilibrium CDE model to inverse hydrogeological parameters of karst conduit[J]. Geotechnical Investigation & Surveying,2014,42(9):56 − 59. (in Chinese with English abstract)
[7] TAYLOR G. The dispersion of matter in turbulent flow through a pipe[J]. Proceedings of the Royal Society of London,1954,223:446 − 468.
[8] HAUNS M,JEANNIN P Y,ATTEIA O. Dispersion,retardation and scale effect in tracer breakthrough curves in karst conduits[J]. Journal of Hydrology,2001,241(3):177 − 193.
[9] ZHAO Xiaoer,CHANG Yong,WU Jichun,et al. Laboratory investigation and simulation of breakthrough curves in karst conduits with pools[J]. Hydrogeology Journal,2017,25(8):2235 − 2250. doi: 10.1007/s10040-017-1626-9
[10] LI Guangquan,LOPER D E,KUNG R. Contaminant sequestration in karstic aquifers:Experiments and quantification[J]. Water Resources Research,2008,44(2):401 − 422.
[11] FAULKNER J,HU B X,KISH S,et al. Laboratory analog and numerical study of groundwater flow and solute transport in a karst aquifer with conduit and matrix domains[J]. Journal of Contaminant Hydrology,2009,110(1/2):34 − 44.
[12] MOHAMMADI Z,GHARAAT M J,FIELD M. The effect of hydraulic gradient and pattern of conduit systems on tracing tests:Bench-scale modeling[J]. Ground Water,2018,57(1):110 − 125.
[13] SHU Longcang,ZOU Zhike,LI Fulin,et al. Laboratory and numerical simulations of spatio-temporal variability of water exchange between the fissures and conduits in a karstic aquifer[J]. Journal of Hydrology,2020,590(11):125219.
[14] WANG Chaoqi,WANG Xiaoguang,MAJDALANI S,et al. Influence of dual conduit structure on solute transport in karst tracer tests:An experimental laboratory study[J]. Journal of Hydrology,2020,590:125255. doi: 10.1016/j.jhydrol.2020.125255
[15] FIELD M S,LEIJ F J. Solute transport in solution conduits exhibiting multi-peaked breakthrough curves[J]. Journal of Hydrology,2012,440/441:26 − 35. doi: 10.1016/j.jhydrol.2012.03.018
[16] 袁道先. 中国西南部的岩溶及其与华北岩溶的对比[J]. 第四纪研究,1992,12(4):352 − 361. [YUAN Daoxian. Karst in Southwest China and its comparison with karst in North China[J]. Quaternary Sciences,1992,12(4):352 − 361. (in Chinese with English abstract)
[17] ZHAO Xiaoer,CHANG Yong,WU Jichun,et al. Investigating the relationships between parameters in the transient storage model and the pool volume in karst conduits through tracer experiments[J]. Journal of Hydrology,2021,593:125825. doi: 10.1016/j.jhydrol.2020.125825
[18] WU Yuexia,HUNKELER D. Hyporheic exchange in a karst conduit and sediment system – A laboratory analog study[J]. Journal of Hydrology,2013,501:125 − 132. doi: 10.1016/j.jhydrol.2013.07.040
[19] MOHAMMADI Z,ILLMAN W A,FIELD M. Review of laboratory scale models of karst aquifers:Approaches,similitude,and requirements[J]. Ground Water,2021,59(2):163 − 174. doi: 10.1111/gwat.13052
[20] 郭芳,陈坤琨,姜光辉. 岩溶地下河沉积物对氨氮的等温吸附特征[J]. 环境科学,2011,32(2):501 − 507. [GUO Fang,CHEN Kunkun,JIANG Guanghui. Characteristic of ammonia nitrogen adsorption on karst underground river sediments[J]. Environmental Science,2011,32(2):501 − 507. (in Chinese with English abstract)
[21] BARBERA J A,MUDARRA M,ANDREO B,et al. Regional-scale analysis of karst underground flow deduced from tracing experiments:Examples from carbonate aquifers in Malaga Province,Southern Spain[J]. Hydrogeology Journal,2018,26(1):23 − 40. doi: 10.1007/s10040-017-1638-5
[22] FIELD M. The QTRACER2 program for tracer-breakthrough curve analysis for tracer tests in karstic aquifers and other hydrologic systems[M]. Washington: US Environmental Protection Agency, 2002.
[23] ZHANG Qing,LUO Shaohe,MA Haichun,et al. Simulation on the water flow affected by the shape and density of roughness elements in a single rough fracture[J]. Journal of Hydrology,2019,573:456 − 468. doi: 10.1016/j.jhydrol.2019.03.069
[24] SCHMADEL N M,WARD A S,KURZ M J,et al. Stream solute tracer timescales changing with discharge and reach length confound process interpretation[J]. Water Resources Research,2016,52(4):3227 − 3245. doi: 10.1002/2015WR018062
[25] 陈余道,程亚平,王恒,等. 岩溶地下河管道流和管道结构及参数的定量示踪—以桂林寨底地下河为例[J]. 水文地质工程地质,2013,40(5):11 − 15. [CHEN Yudao,CHENG Yaping,WANG Heng,et al. Quantitative tracing study of hydraulic and geometric parameters of a karst underground river:Exemplified by the Zhaidi Underground River in Gulin[J]. Hydrogeology & Engeneering Geology,2013,40(5):11 − 15. (in Chinese with English abstract)
[26] BENCALA,K E,WALTERS R A. Simulation of solute transport in a mountain pool-and-riffle stream a transient storage model[J]. Water Resources Research,1983,19(3):718 − 724. doi: 10.1029/WR019i003p00718
[27] RUNKEL R L. One-dimensional transport with inflow and storage (OTIS): A solute transport model for streams and rivers[R]. Reston: US Geological Survey, 1998.
[28] WAGNER B J,HARVEY J W. Experimental design for estimating parameters of rate-limited mass transfer:Analysis of stream tracer studies[J]. Water Resources Research,1997,33(7):1731 − 1741. doi: 10.1029/97WR01067
[29] SCHMID B H. Simplification in longitudinal transport modeling:Case of instantaneous slug releases[J]. Journal of Hydrologic Engineering,2004,9(4):319 − 324. doi: 10.1061/(ASCE)1084-0699(2004)9:4(319)
[30] SCHMID,B H,INNOCENTI I,SANFILIPPO U. Characterizing solute transport with transient storage across a range of flow rates:The evidence of repeated tracer experiments in Austrian and Italian streams[J]. Advances in Water Resources,2010,33(11):1340 − 1346. doi: 10.1016/j.advwatres.2010.06.001
[31] GOLDSCHEIDER N. A new quantitative interpretation of the long-tail and plateau-like breakthrough curves from tracer tests in the artesian karst aquifer of Stuttgart,Germany[J]. Hydrogeology Journal,2008,16(7):1311 − 1317. doi: 10.1007/s10040-008-0307-0
[32] 陈亚洲,董维红. 利用示踪试验时间-浓度曲线分析岩溶管道结构特征[J]. 水文地质工程地质,2022,49(1):41 − 47. [CHEN Yazhou,DONG Weihong. Analysis of structural characteristics of karst conduit by time-concentration curve of tracer test[J]. Hydrogeology & Engineering Geology,2022,49(1):41 − 47. (in Chinese with English abstract)
[33] 赵一,李衍青,覃星铭,等. 南洞地下河岩溶管道展布及结构特征的示踪试验解析[J]. 中国岩溶,2017,36(2):226 − 233. [ZHAO Yi,LI Yanqing,QIN Xingming,et al. Tracer tests on distribution and structural characteristics of karst channels in Nandong underground river drainage[J]. Carsologica Sinica,2017,36(2):226 − 233. (in Chinese with English abstract)
[34] 谢国文,杨平恒,卢丙清,等. 基于高分辨率示踪技术的岩溶隧道涌水来源识别及含水介质研究[J]. 中国岩溶,2018,37(6):892 − 899. [XIE Guowen,YANG Pingheng,LU Bingqing,et al. Application of high-resolution tracer technique in identifying the source of water gushing and the structure of aquifer medium in karst tunnel[J]. Carsologica Sinica,2018,37(6):892 − 899. (in Chinese with English abstract)
-



 下载:
下载: