Effect of injection time and hydrostatic pressure on chloride migration in a porous geothermal reservoir
-
摘要:
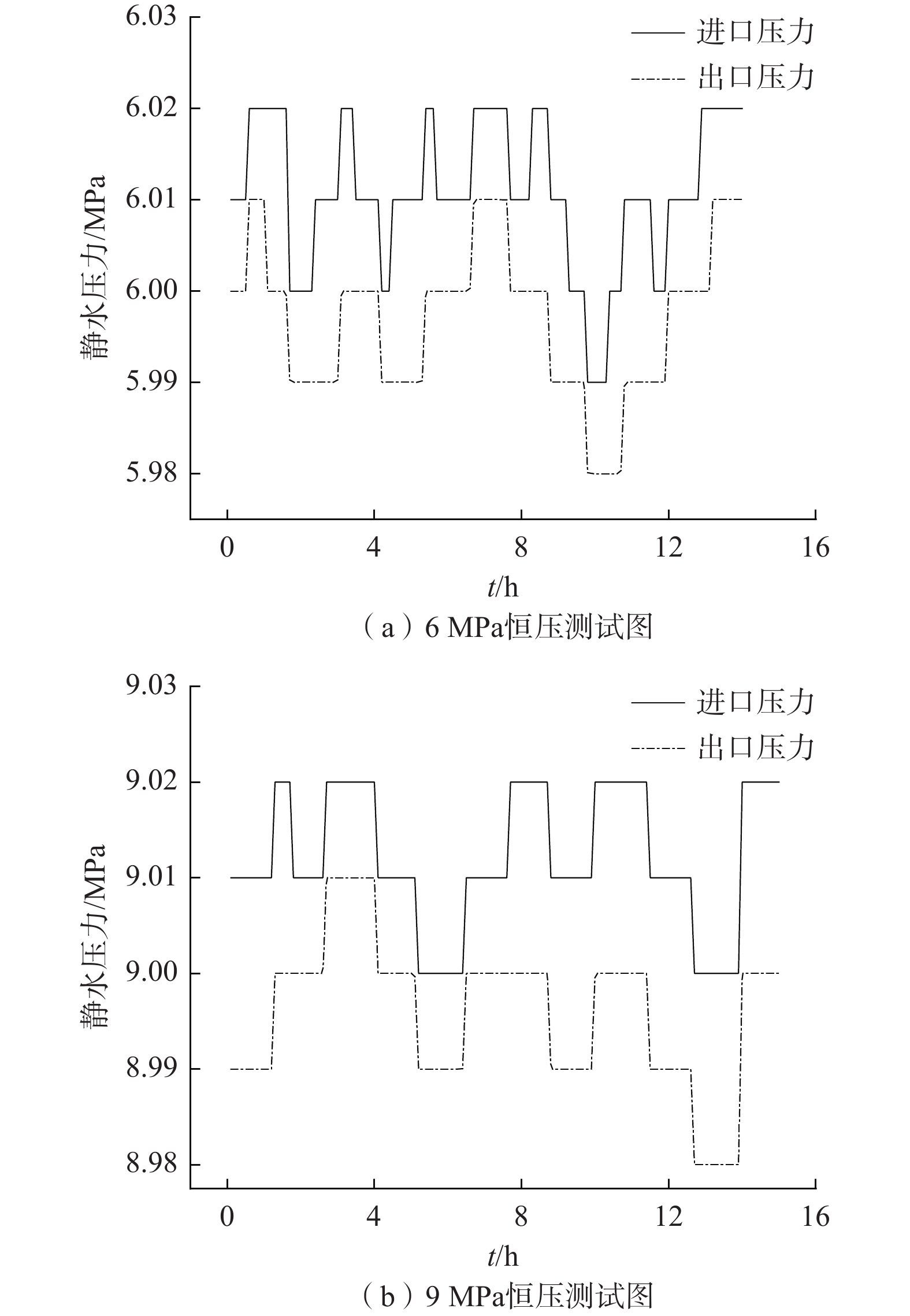
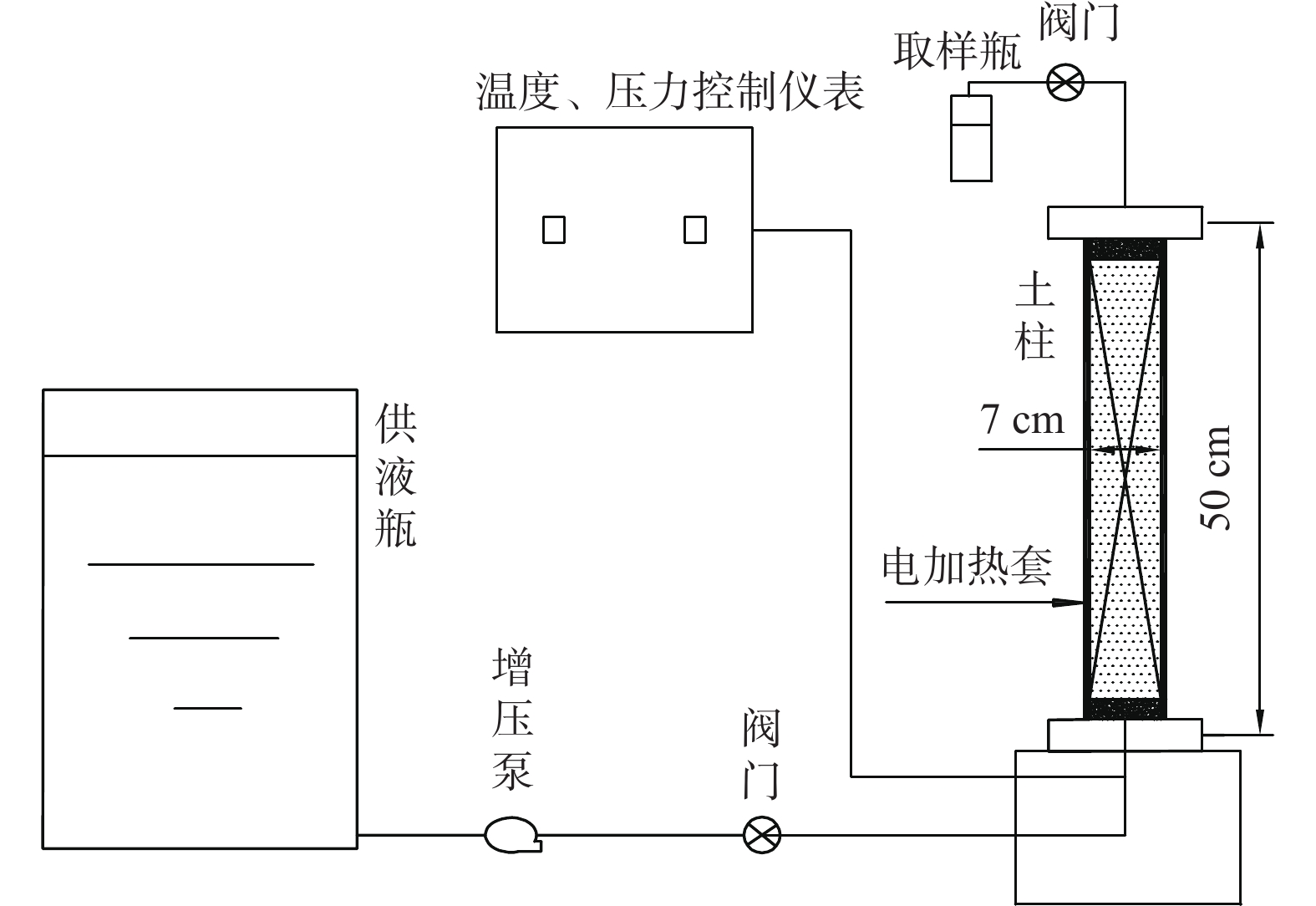
迄今为止,注入时间和静水压力对溶质在深层承压地热水中的运移规律影响研究少有报道。通过模拟35℃的低温地热环境,开展了注入时间1,2,3,4,5 h以及静水压力0,6,9 MPa条件下Cl−的运移柱模拟试验。采用CXTFIT 2. 1软件进行数值模拟,探讨了孔隙型热储砂土中Cl−的运移规律和影响因素。结果表明:在模拟的低温孔隙型热储层中,不同注入时间和静水压力下,Cl−的运移曲线均呈正态对称分布,一维对流弥散(CDE)模型也可较好地表征其穿透曲线,因此溶质扩散过程符合菲克定律。注入时间的不同,会引起Cl−的穿透曲线、运移参数发生变化,这与不同注入时间条件下溶质注入总量、柱内溶质浓度差以及分子扩散能力不同有关。在不同静水压力条件下,弥散系数从0 MPa的25.22 cm2/h增加到9 MPa的36.13 cm2/h,分子扩散系数、机械弥散系数以及弥散度也随之增大,因此溶质的弥散作用随静水压力的增大而增强。研究结果对于丰富地下水的溶质运移理论具有重要意义。
Abstract:Few studies have focused on the effects of injection time and hydrostatic pressure on the solute transport in porous geothermal reservoirs to date. The chloride displacement experiments were individually carried out at 35 °C at the injection time of 1 h, 2 h, 3 h, 4 h and 5 h individually through the simulated columns packed with the thermal reservoir fine sand. Column experiments were performed at 35 °C at hydrostatic pressure of 0, 6 and 9 MPa individually. By using the one-dimensional CDE model in the CXTFIT 2.1 software, the migration law of Cl− and its influencing factors in the studied matrix were examined. The results show that the Cl− breakthrough curves under different injection time and hydrostatic pressure are symmetrically distributed, and they can all be well described by the CDE model. Thus, the solute dispersion can conform to the Fick’s law in the simulated low-temperature pore geothermal water. The breakthrough curve and transport parameters of Cl− are highly correlated with the injection time due to the variations of the total amount of solute mass injected, concentration differences and molecular diffusion ability in the studied geothermal water. In addition, the value of D increases from 25.22 cm2/h at 0 MPa to 36.13 cm2/h at 9 MPa, combining with the increasing molecular diffusion coefficient, dispersion coefficient and dispersivity with hydrostatic pressure. Hence, the solute hydrodynamic dispersion in the simulated sandy column are enhanced with the increasing hydrostatic pressure. The results are of great significance to enrich the theory of solute transport in groundwater.
-
Key words:
- low temperature pore geothermal water /
- injection time /
- hydrostatic pressure /
- chloride /
- migration law
-

-
表 1 岩样的物理性质
Table 1. Physical properties of rock samples
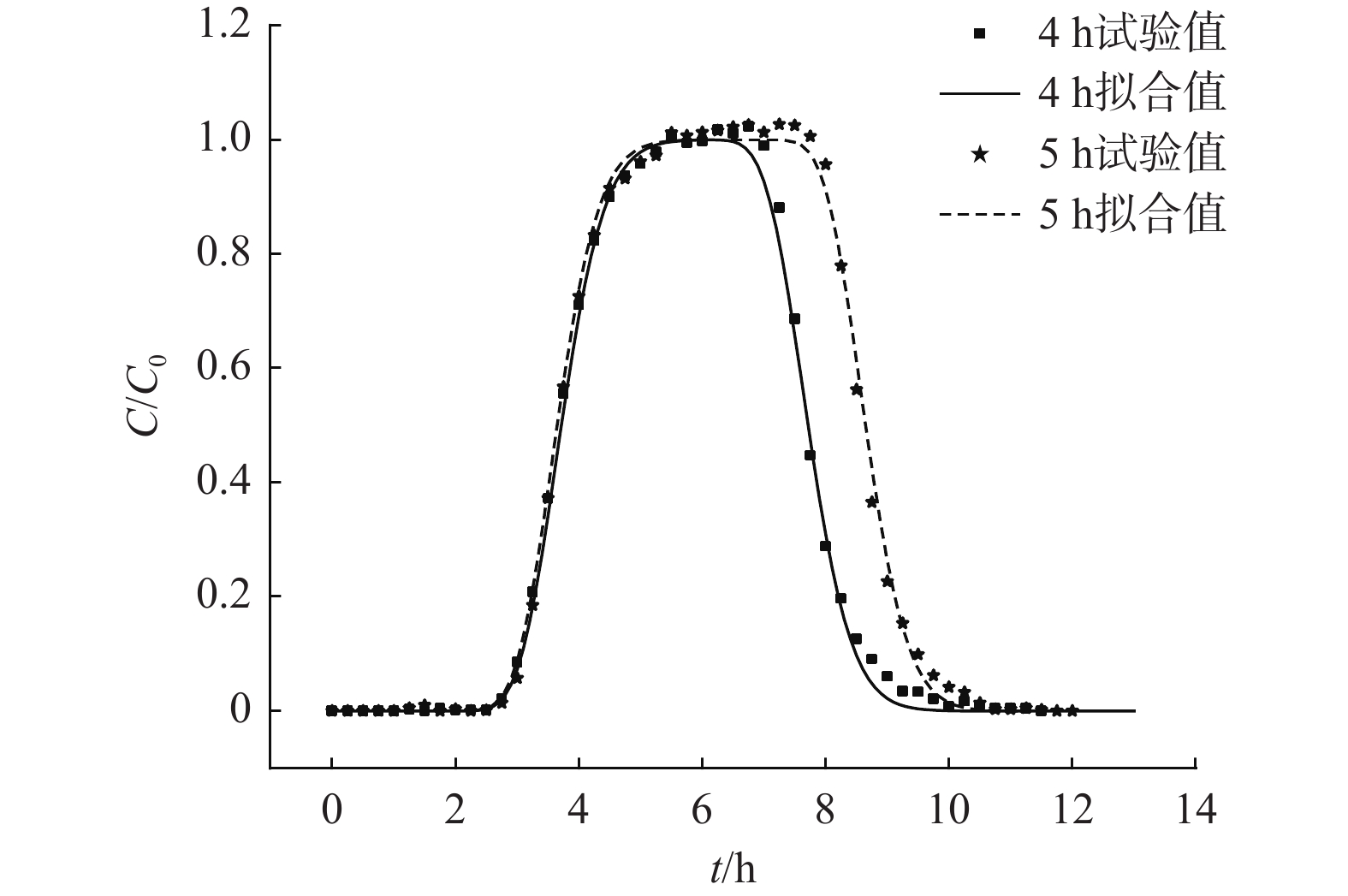
测定指标 数值 密度/(g·cm−3) 2.52 吸湿水/% 2.78 含水率/% 13.15 pH(水土比2.5∶1) 8.60~8.90 表 2 不同注入时间Cl−穿透曲线CDE拟合参数值
Table 2. CDE fitting parameters of Cl- penetration curve at different injection times
注入时间
/h达西流速
/(cm·h−1)填充土柱的
孔隙度CDE模型 弥散度/cm 机械弥散系数
/(cm2·h−1)Peclet数 分子扩散系数
/(cm2·h−1)纵向弥散系数
/(cm2·h−1)孔隙流速
/(cm· h−1)R2 1 6.24 0.45 10.68 12.88 0.981 0.829 10.637 60.3 0.043 2 11.28 12.1 0.99 0.932 11.235 53.635 0.045 3 25.22 15.03 0.996 1.678 25.119 29.798 0.101 4 7.221 13.31 0.997 0.542 7.191 92.162 0.029 5 7.097 13.57 0.998 0.523 7.069 95.604 0.028 注:λ = DL/V;Dh =DL−Df;Pe=VL/DL;Df =Vd / Pe。其中,λ ——弥散度/cm;Df——分子扩散系数/(cm2·h−1);Dh——机械弥散系数/(cm2·h−1);Pe——Peclet 数;d——填充砂土的平均粒径/cm,取0.2 cm;L——柱子长度/cm,取50 cm。 表 3 不同静水压力Cl−穿透曲线CDE拟合参数值
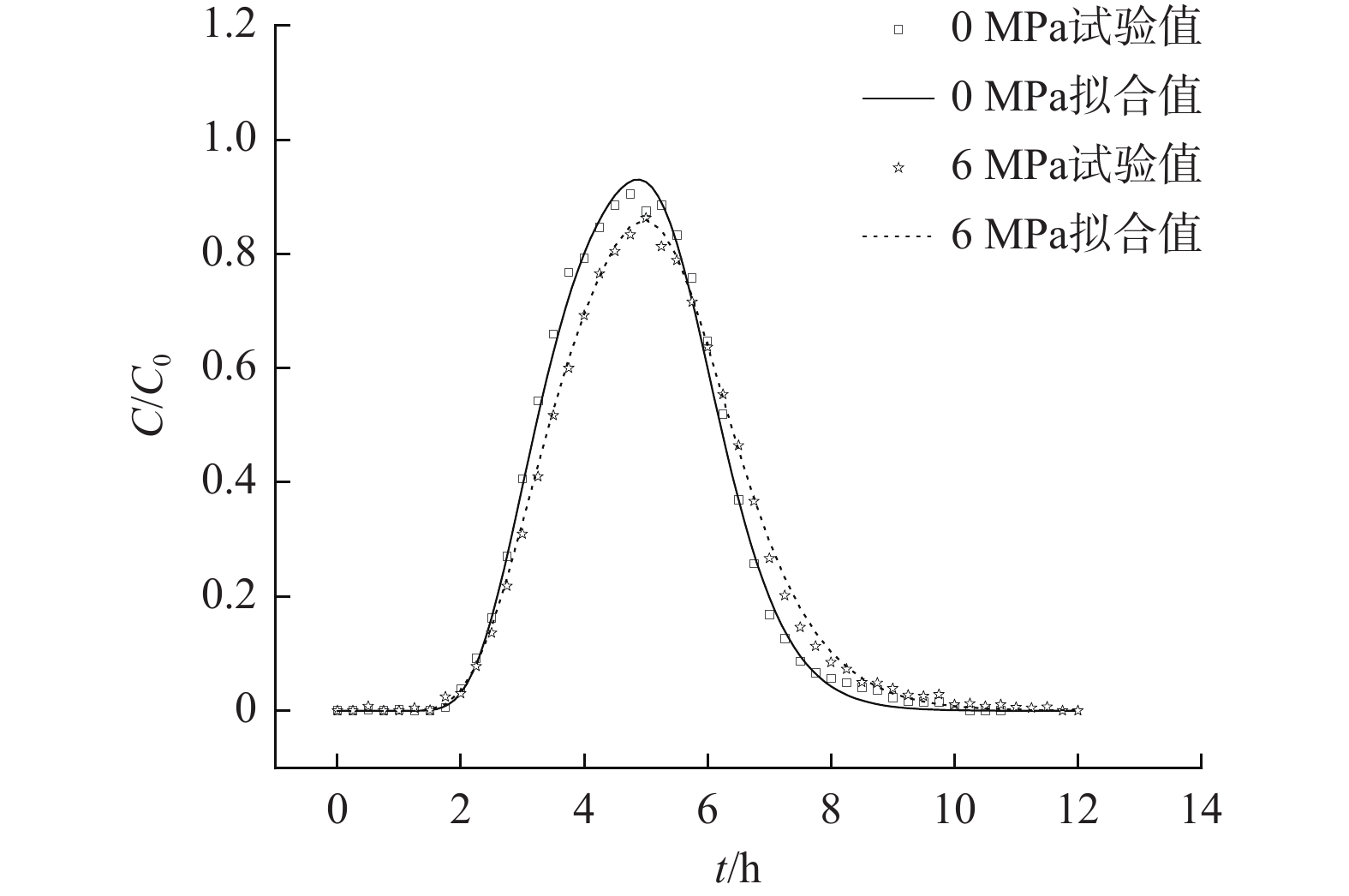
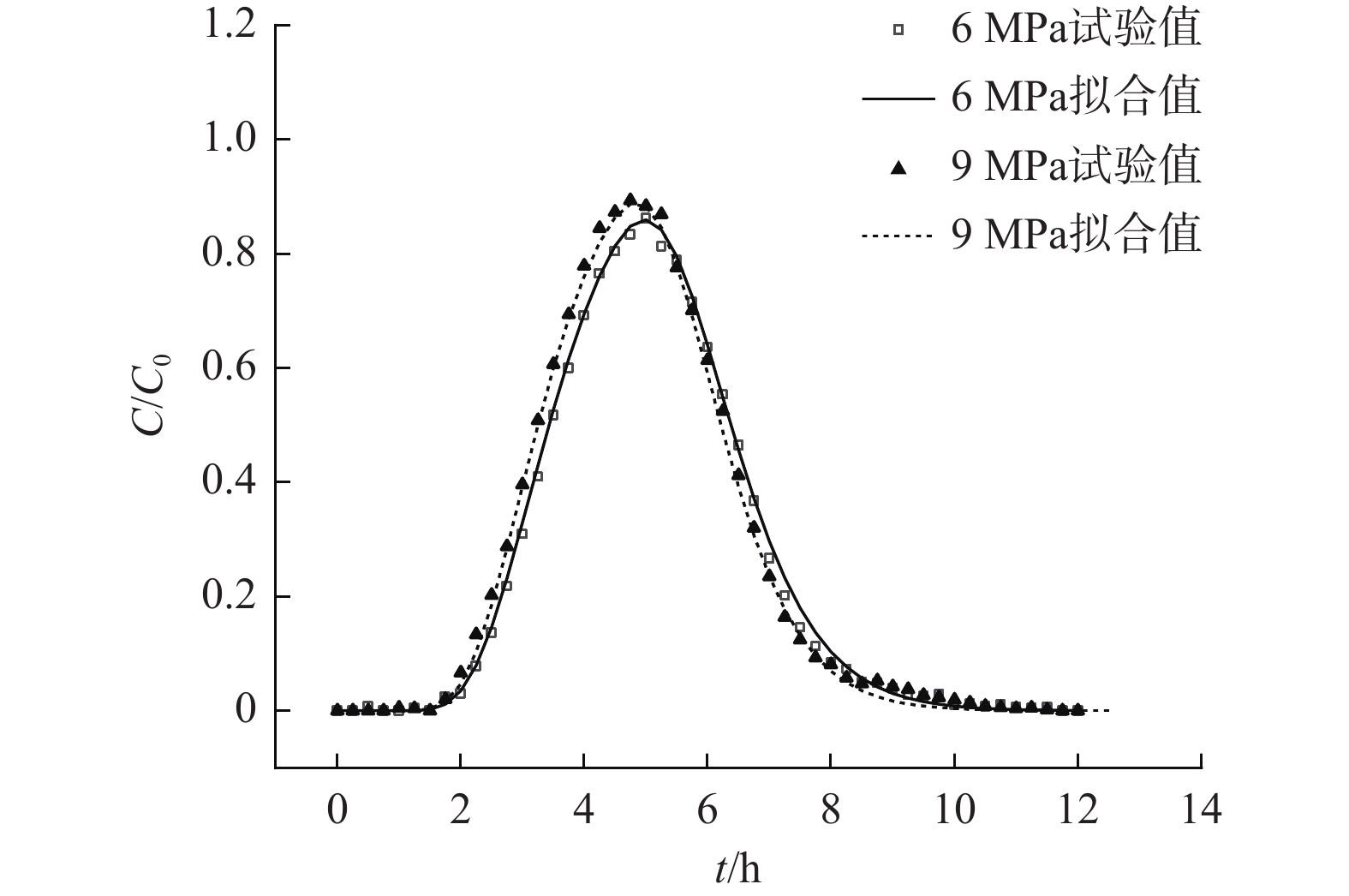
Table 3. CDE fitting parameters of Cl- penetration curves under different hydrostatic pressures
静水压力
/MPa达西流速
/(cm·h−1)填充土柱的
孔隙度CDE模型 弥散度
/cm机械弥散系数
/(cm2·h−1)Peclet数 分子扩散系数
/(cm2·h−1)纵向弥散系数/(cm2·h−1) R2 0
6.24
0.4525.22 0.996 1.678 25.119 29.798 0.101 6 32.29 0.998 2.316 32.161 21.586 0.129 9 36.13 0.994 2.38 35.985 21.007 0.145 -
[1] 朱菊艳,郭海朋,李文鹏,等. 华北平原地面沉降与深层地下水开采关系[J]. 南水北调与水利科技,2014,12(3):165 − 169. [ZHU Juyan,GUO Haipeng,LI Wenpeng,et al. Relationship between land subsidence and deep groundwater yield in the North China Plain[J]. South-to-North Water Transfers and Water Science & Technology,2014,12(3):165 − 169. (in Chinese with English abstract)
[2] WANG Xinyi,ZHAO Li,LIU Xiaoman,et al. Temperature effect on the transport of nitrate and ammonium ions in a loose-pore geothermal reservoir[J]. Journal of Geochemical Exploration,2013,124:59 − 66. doi: 10.1016/j.gexplo.2012.08.008
[3] JIANG Lei,BAI Li,ZHAO Yan,et al. Combining InSAR and hydraulic head measurements to estimate aquifer parameters and storage variations of confined aquifer system in Cangzhou,North China plain[J]. Water Resources Research,2018,54(10):8234 − 8252. doi: 10.1029/2017WR022126
[4] BAI Lin,JIANG Liming,ZHAO Yong,et al. Quantifying the influence of long-term overexploitation on deep groundwater resources across Cangzhou in the North China Plain using InSAR measurements[J]. Journal of Hydrology,2022,605:127368. doi: 10.1016/j.jhydrol.2021.127368
[5] 马致远,侯晨,席临平,等. 超深层孔隙型热储地热尾水回灌堵塞机理[J]. 水文地质工程地质,2013,40(5):133 − 139. [MA Zhiyuan,HOU Chen,XI Linping,et al. Reinjection clogging mechanism of used geothermal water in a super-deep-porous reservoir[J]. Hydrogeology & Engineering Geology,2013,40(5):133 − 139. (in Chinese with English abstract) doi: 10.16030/j.cnki.issn.1000-3665.2013.05.029
[6] 赵亚洲, 庞忠和, 马智博, 等. 地热储多场多相数值模拟与介尺度技术进展[J]. 科技促进发展, 2020, 16(增刊1): 359 − 366
ZHAO Yazhou, PANG Zhonghe, MA Zhibo, et al. Advance research on multi-field and multi-phase simulation of geothermal reservoir[J]. Science & Technology for Development, 2020, 16(Sup1): 359 − 366. (in Chinese with English abstract)
[7] NIELSEN K K,NELLIS G F,KLEIN S A. Numerical modeling of the impact of regenerator housing on the determination of Nusselt numbers[J]. International Journal of Heat and Mass Transfer,2013,65:552 − 560. doi: 10.1016/j.ijheatmasstransfer.2013.06.032
[8] BHATTACHARYA P,JACKS G,AHMED K M,et al. Arsenic in groundwater of the Bengal delta plain aquifers in Bangladesh[J]. Bulletin of Environmental Contamination and Toxicology,2002,69(4):538 − 545. doi: 10.1007/s00128-002-0095-5
[9] WANG Quanrong,GU Haochen,ZHAN Hongbin,et al. Mixing effect on reactive transport in a column with scale dependent dispersion[J]. Journal of Hydrology,2020,582:124494. doi: 10.1016/j.jhydrol.2019.124494
[10] 黄健,单慧媚,彭三曦,等. 水动力因素对砷在河砂中迁移影响的柱试验与模拟[J]. 水文地质工程地质,2022,49(2):195 − 206. [HUANG Jian,SHAN Huimei,PENG Sanxi,et al. Influence of hydrodynamic factors on the migration of arsenic in river sand:column experiment and models[J]. Hydrogeology & Engineering Geology,2022,49(2):195 − 206. (in Chinese with English abstract) doi: 10.16030/j.cnki.issn.1000-3665.202105016
[11] 陈星欣, 白冰, 于涛, 等. 粒径和渗流速度对多孔介质中悬浮颗粒迁移和沉积特性的耦合影响[J]. 岩石力学与工程学报, 2013, 32(增刊1): 2840 − 2845
CHEN Xingxin, BAI Bing, YU Tao, et al. Coupled effects of particle size and flow rate on characteristics of particle transportation and deposition in porous media[J]. Chinese Journal of Rock Mechanics and Engineering, 2013, 32(Sup1): 2840 − 2845. (in Chinese with English abstract)
[12] 曹孟雄,陈刚,胡成. 初始浓度分布对一维含水层中溶质运移的影响[J]. 中国环境科学,2021,41(5):2226 − 2231. [CAO Mengxiong,CHEN Gang,HU Cheng. Impact of initial concentration distribution on solute transport in the one-dimensional aquifer[J]. China Environmental Science,2021,41(5):2226 − 2231. (in Chinese with English abstract) doi: 10.19674/j.cnki.issn1000-6923.2021.0234
[13] BOND W J,WIERENGA P J. Immobile water during solute transport in unsaturated sand columns[J]. Water Resources Research,1990,26(10):2475 − 2481. doi: 10.1029/WR026i010p02475
[14] PADILLA I Y,YEH T C J,CONKLIN M H. The effect of water content on solute transport in unsaturated porous media[J]. Water Resources Research,1999,35(11):3303 − 3313. doi: 10.1029/1999WR900171
[15] ZAHEER M,WEN Zhang,ZHAN Hongbin,et al. An experimental study on solute transport in one-dimensional clay soil columns[J]. Geofluids,2017,2017:1 − 17.
[16] WU Mingchang,HSIEH P C. Analytical modeling of solute transport in a two-zoned porous medium flow[J]. Water,2022,14(3):323. doi: 10.3390/w14030323
[17] JIN Guangqiu,HAO Yongfei,WEI Jie,et al. The effect of density-driven flow on the transport of high-concentration solutes in the hyporheic zone[J]. Hydrological Processes,2021,35(1):e13949.
[18] 赵丽,张庆,刘小满,等. 地热环境下
$ {\rm{NO}}_3^- $ $ {\rm{NO}}_3^- $ [19] 张庆,赵丽,王心义.
$ {\rm{NH}}_4^+ $ $ {\rm{NH}}_4^+ $ [20] 林学钰,王心义,廖资生. 地下热流系统及其开发工程配置—以河南省开封市区为例[J]. 吉林大学学报(地球科学版),2008,38(6):913 − 919. [LIN Xueyu,WANG Xinyi,LIAO Zisheng. The geothermal fluid system and its exploitation engineering scheme:Taking Kaifeng area,Henan Province as example[J]. Journal of Jilin University (Earth Science Edition),2008,38(6):913 − 919. (in Chinese with English abstract)
[21] 齐玉峰,王现国,王关杰,等. 开封凹陷区地热资源开发利用与保护[J]. 地下水,2007,29(4):77 − 79. [QI Yufeng,WANG Xianguo,WANG Guanjie,et al. Development and protection of geothermal resources in Kaifeng depression[J]. Ground Water,2007,29(4):77 − 79. (in Chinese with English abstract) doi: 10.3969/j.issn.1004-1184.2007.04.028
[22] NEUMAN S P,TARTAKOVSKY D M. Perspective on theories of non-Fickian transport in heterogeneous media[J]. Advances in Water Resources,2009,32(5):670 − 680. doi: 10.1016/j.advwatres.2008.08.005
[23] LANGEVIN C D,GUO Weixing. MODFLOW/MT3DMS-based simulation of variable-density ground water flow and transport[J]. Ground Water,2006,44(3):339 − 351. doi: 10.1111/j.1745-6584.2005.00156.x
[24] WOOD M,SIMMONS C T,HUTSON J L. A breakthrough curve analysis of unstable density-driven flow and transport in homogeneous porous media[J]. Water Resources Research,2004,40(3):1 − 9.
[25] SIMMONS C T,FENSTEMAKER T R,SHARP J M J. Variable-density groundwater flow and solute transport in heterogeneous porous media:Approaches,resolutions and future challenges[J]. Journal of Contaminant Hydrology,2001,52(1/2/3/4):245 − 275.
[26] 张建桥, 窦智, 张学羿. 多孔介质粒径变异系数对污染物运移的影响[J/OL]. 地球科学. Http://Kns.cnki.net/Kcms/setail/42.1874.p.200211101.1909.016.html.
ZHANG Jianqiao, DOU Zhi, ZHANG Xueyi. Effect of coefficient of variation of particle size of porous media on contaminant transport[J/OL]. Earth Science. Http://Kns.cnki.net/Kcms/setail/42.1874.p.200211101.1909.016.html. (in Chinese with English abstract)
[27] 周鸿翔,郑延丰,吴劳生,等. 孔隙尺度多孔介质流体流动与溶质运移高性能模拟[J]. 水科学进展,2020,31(3):422 − 432. [ZHOU Hongxiang,ZHENG Yanfeng,WU Laosheng,et al. Pore-scale simulations of fluid flow and solute transport in porous media by high-performance Lattice Boltzmann Method[J]. Advances in Water Science,2020,31(3):422 − 432. (in Chinese with English abstract) doi: 10.14042/j.cnki.32.1309.2020.03.012
[28] 张兴昊,林丹彤,胡黎明. 基于等效孔隙网络模型的水动力弥散数值模拟[J]. 清华大学学报(自然科学版),2022,62(12):1906 − 1914. [ZHANG Xinghao,LIN Dantong,HU Liming. Numerical simulations of hydrodynamic dispersion based on an equivalent pore network model[J]. Journal of Tsinghua University (Science and Technology),2022,62(12):1906 − 1914. (in Chinese with English abstract)
[29] SIMMONS C T,PIERINI M L,HUTSON J L. Laboratory investigation of variable-density flow and solute transport in unsaturated-saturated porous media[J]. Transport in Porous Media,2002,47(2):215 − 244. doi: 10.1023/A:1015568724369
[30] YU Chuang,WANG Hui,WU Zexiang,et al. Analytical solution for pollutant diffusion in soils with time-dependent dispersion coefficient[J]. International Journal of Geomechanics,2019,19(10):04019109. doi: 10.1061/(ASCE)GM.1943-5622.0001483
[31] 叶逾,蔡芳敏,谢一凡,等. 系统维度对变密度溶质运移的影响研究[J]. 水文地质工程地质,2022,49(1):146 − 153. [YE Yu,CAI Fangmin,XIE Yifan,et al. Effect of the system dimensionality on variable-density solute transport[J]. Hydrogeology & Engineering Geology,2022,49(1):146 − 153. (in Chinese with English abstract) doi: 10.16030/j.cnki.issn.1000-3665.202103015
[32] 苑绍东, 张文杰, 袁姗姗. 溶质运移的弥散度取值试验研究[J]. 岩土力学, 2020(增刊2): 1 − 6
YUAN Shaodong, ZHANG Wenjie, YUAN Shanshan. Dispersion values in solute migration tests[J]. Rock and Soil Mechanics, 2020, (Sup2): 1 − 6. (in Chinese with English abstract)
[33] 张小文,袁伟,王川. 吉林省长山水源地主要含水层野外弥散试验研究[J]. 城市地质,2020,15(4):394 − 403. [ZHANG Xiaowen,YUAN Wei,WANG Chuan. Field dispersion test of main aquifers in Changshan water source area of Jilin Province[J]. Urban Geology,2020,15(4):394 − 403. (in Chinese with English abstract) doi: 10.3969/j.issn.1007-1903.2020.04.007
[34] 袁伟,云智汉. 承压含水层野外弥散试验实施方法研究—以吉林某水源地为例[J]. 城市地质,2019,14(3):74 − 80. [YUAN Wei,YUN Zhihan. Study on implementation method of field dispersion test for confined aquifer:Taking a water source area in Jilin as an example[J]. Urban Geology,2019,14(3):74 − 80. (in Chinese with English abstract) doi: 10.3969/j.issn.1007-1903.2019.03.012
[35] KHUZHAYOROV B,USMONOV A,LONG N M A,et al. Anomalous solute transport in a cylindrical two-zone medium with fractal structure[J]. Applied Sciences,2020,10(15):5349. doi: 10.3390/app10155349
[36] 饶登宇,白冰. 溶质运移中多孔介质弥散度影响因素的 SPH模拟研究[J]. 水利学报,2019,50(7):824 − 834. [RAO Dengyu,BAI Bing. Study on the factors affecting dispersity of porous media by SPH simulation in solute transport[J]. Journal of Hydraulic Engineering,2019,50(7):824 − 834. (in Chinese with English abstract)
[37] 刘春卿,杨劲松,陈小兵,等. 石河子垦区灌耕灰漠土饱和-非饱和土壤水动力弥散试验研究[J]. 灌溉排水学报,2008,27(1):39 − 42. [LIU Chunqing,YANG Jinsong,CHEN Xiaobing,et al. Hydrodynamic dispersion coefficient of saturated-unsaturated soil in Shihezi irrigation areas[J]. Journal of Irrigation and Drainage,2008,27(1):39 − 42. (in Chinese with English abstract) doi: 10.13522/j.cnki.ggps.2008.01.020
[38] 任长江,王建华,赵勇,等. 改进的水动力参数模型在非饱和土壤溶质运移问题中的应用[J]. 水动力学研究与进展A辑,2018,33(4):480 − 489. [REN Changjiang,WANG Jianhua,ZHAO Yong,et al. Application of improved hydrodynamic parameters model for solute transport problem in unsaturation soil[J]. Chinese Journal of Hydrodynamics,2018,33(4):480 − 489. (in Chinese with English abstract) doi: 10.16076/j.cnki.cjhd.2018.04.009
-



 下载:
下载: