A study of the law of groundwater seepage movement in a confined aquifer under seismic waves
-
摘要:
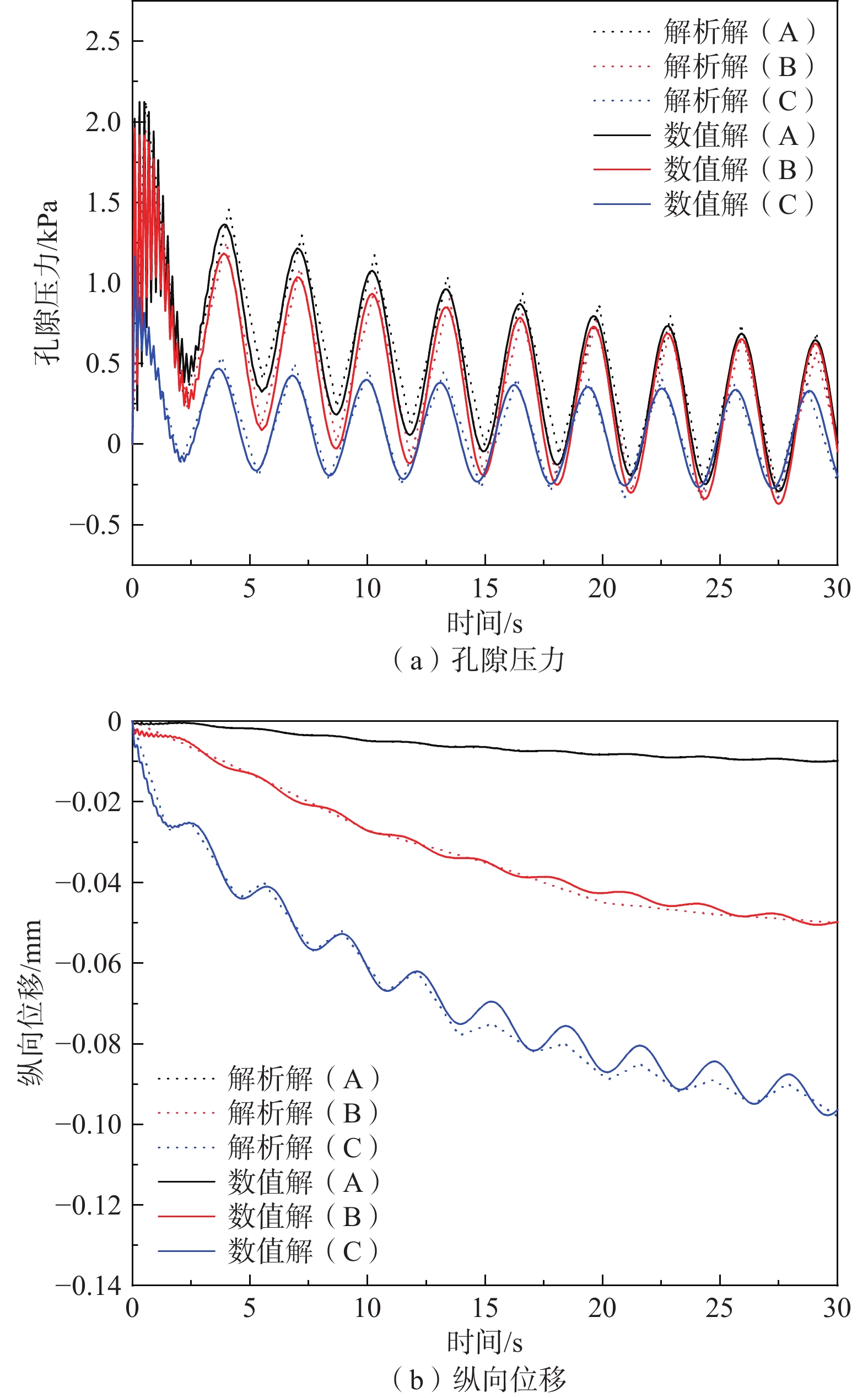
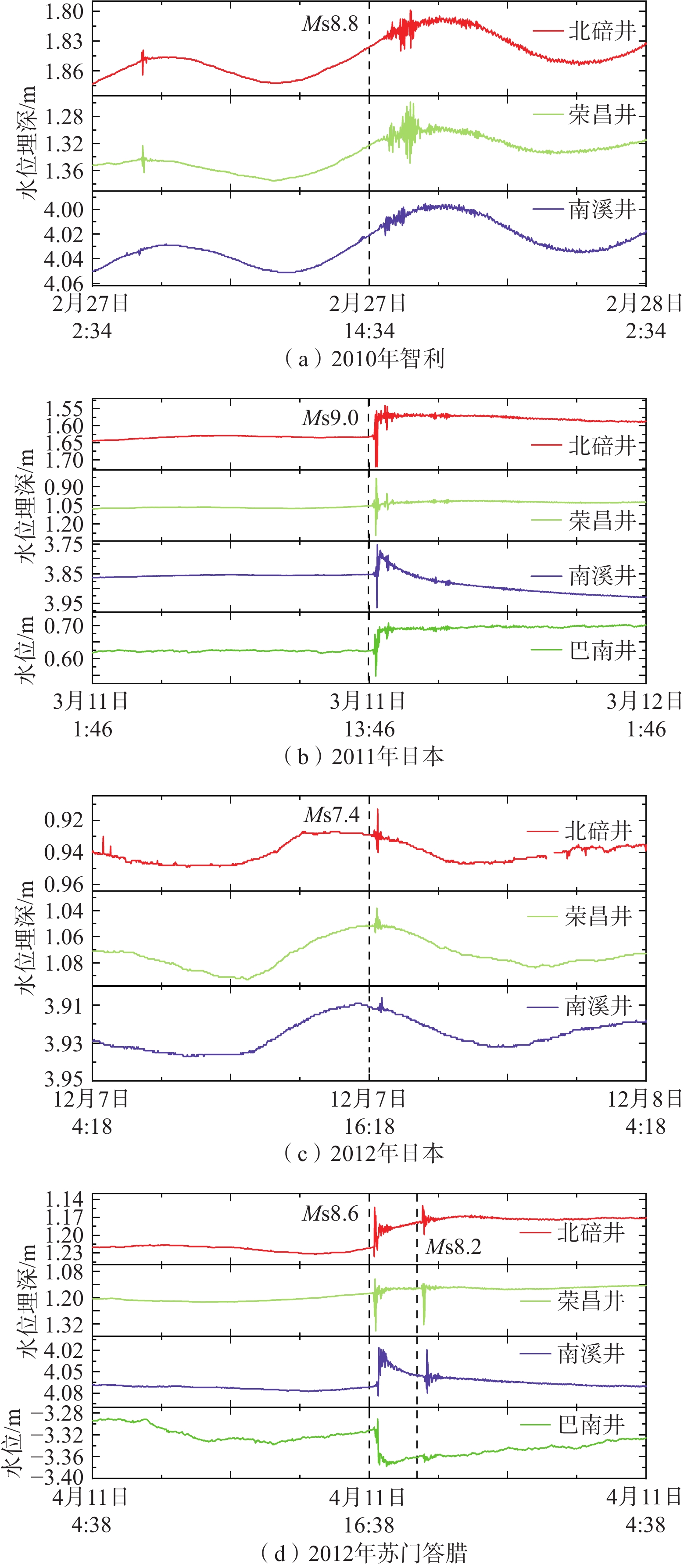
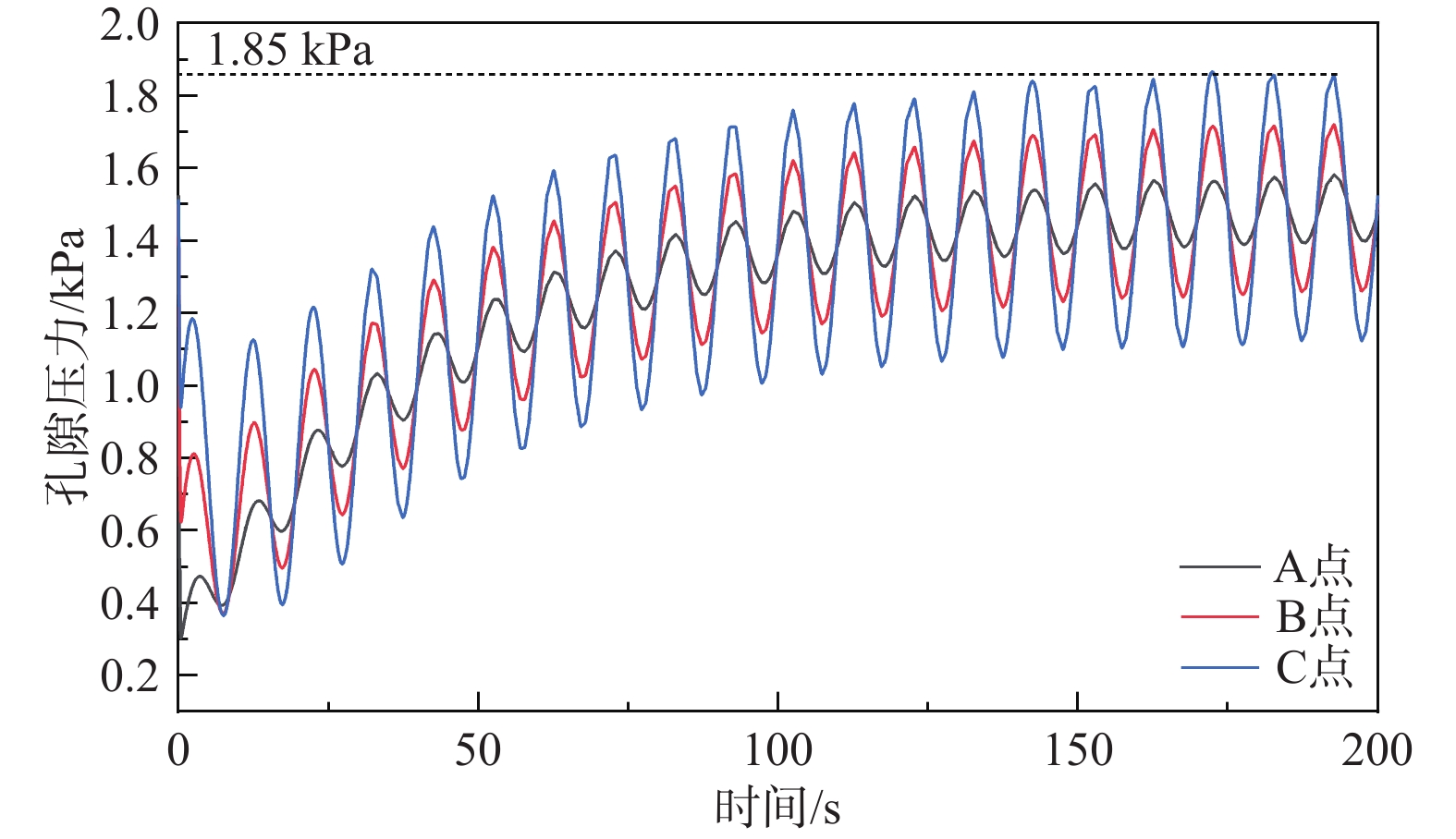
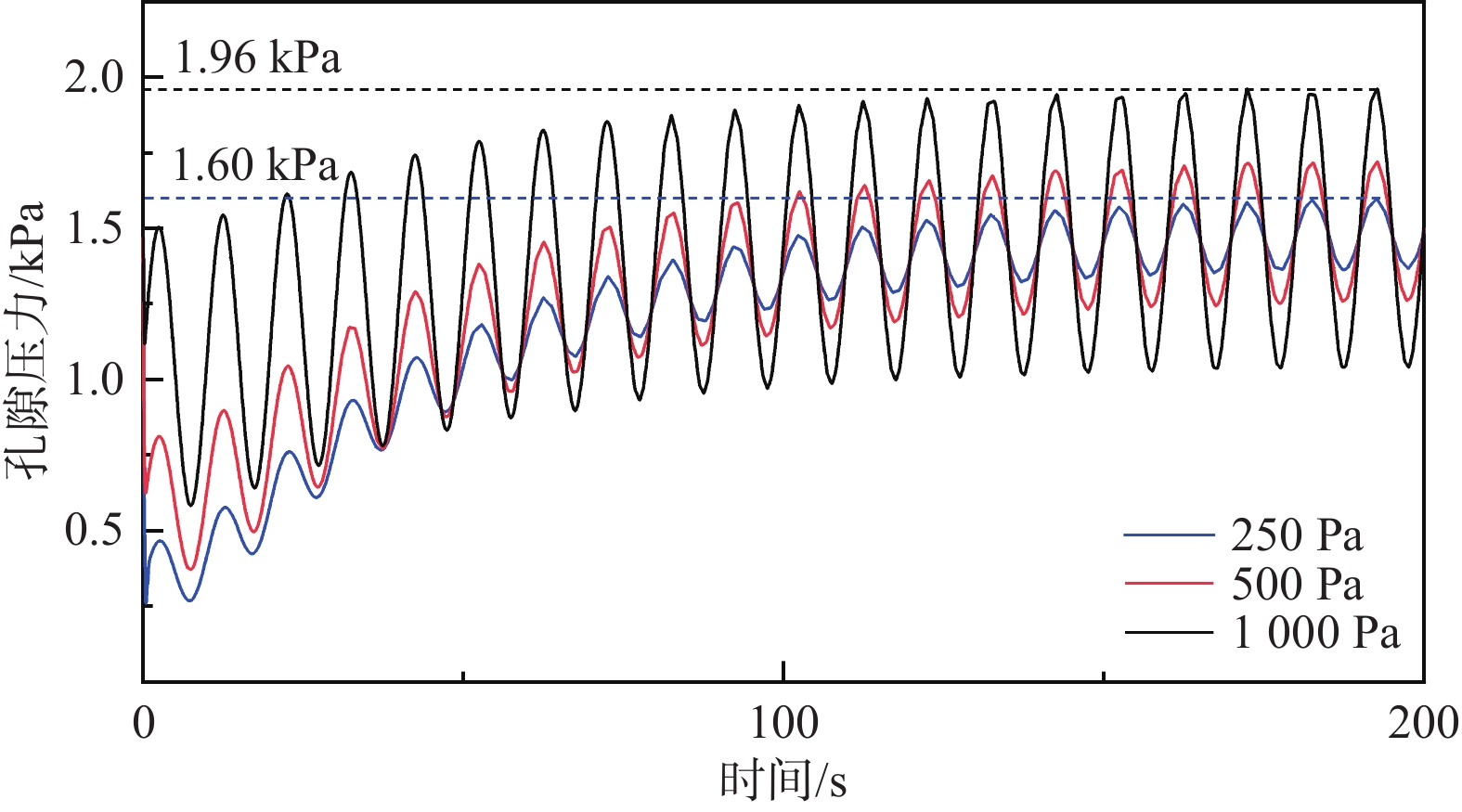
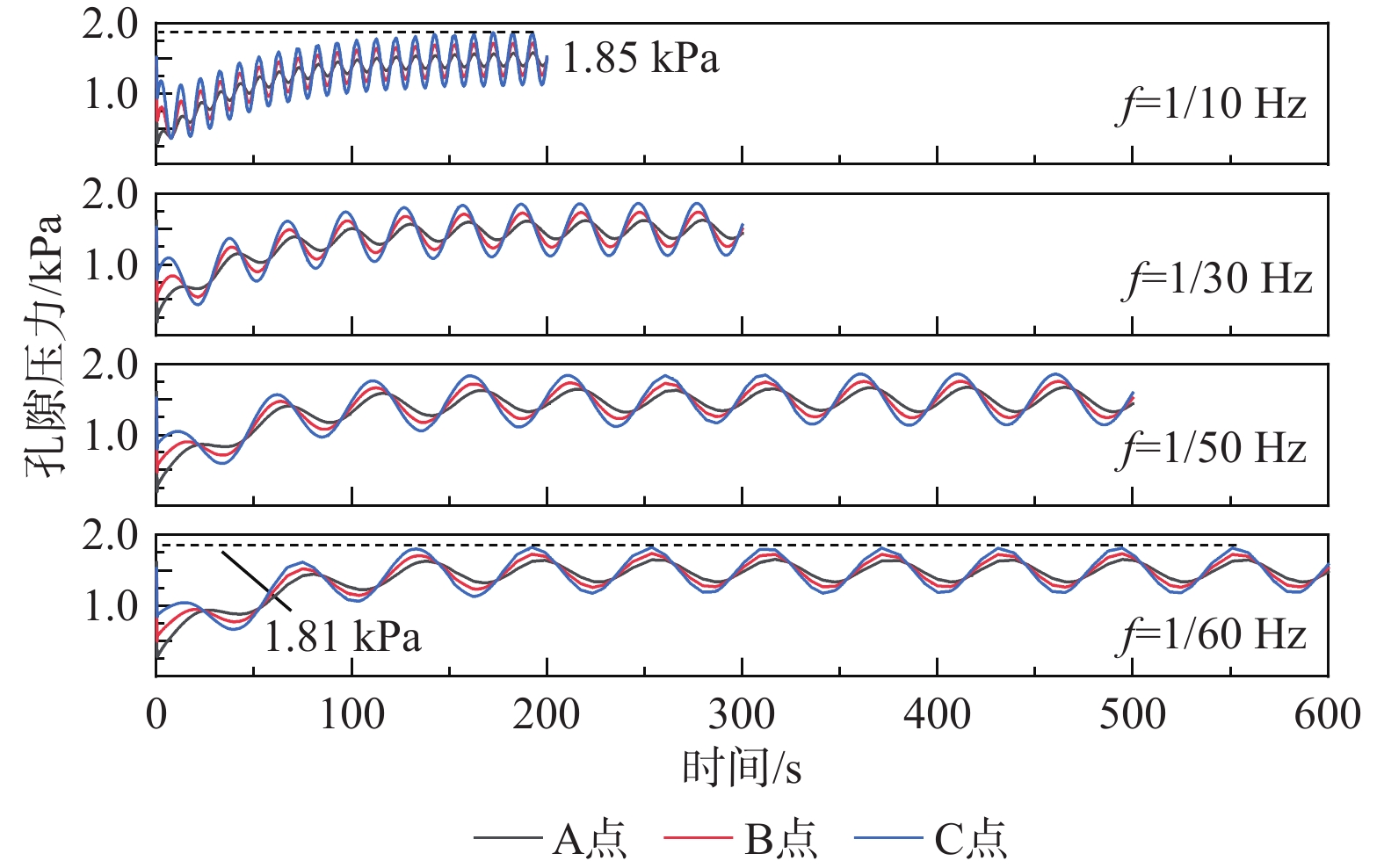
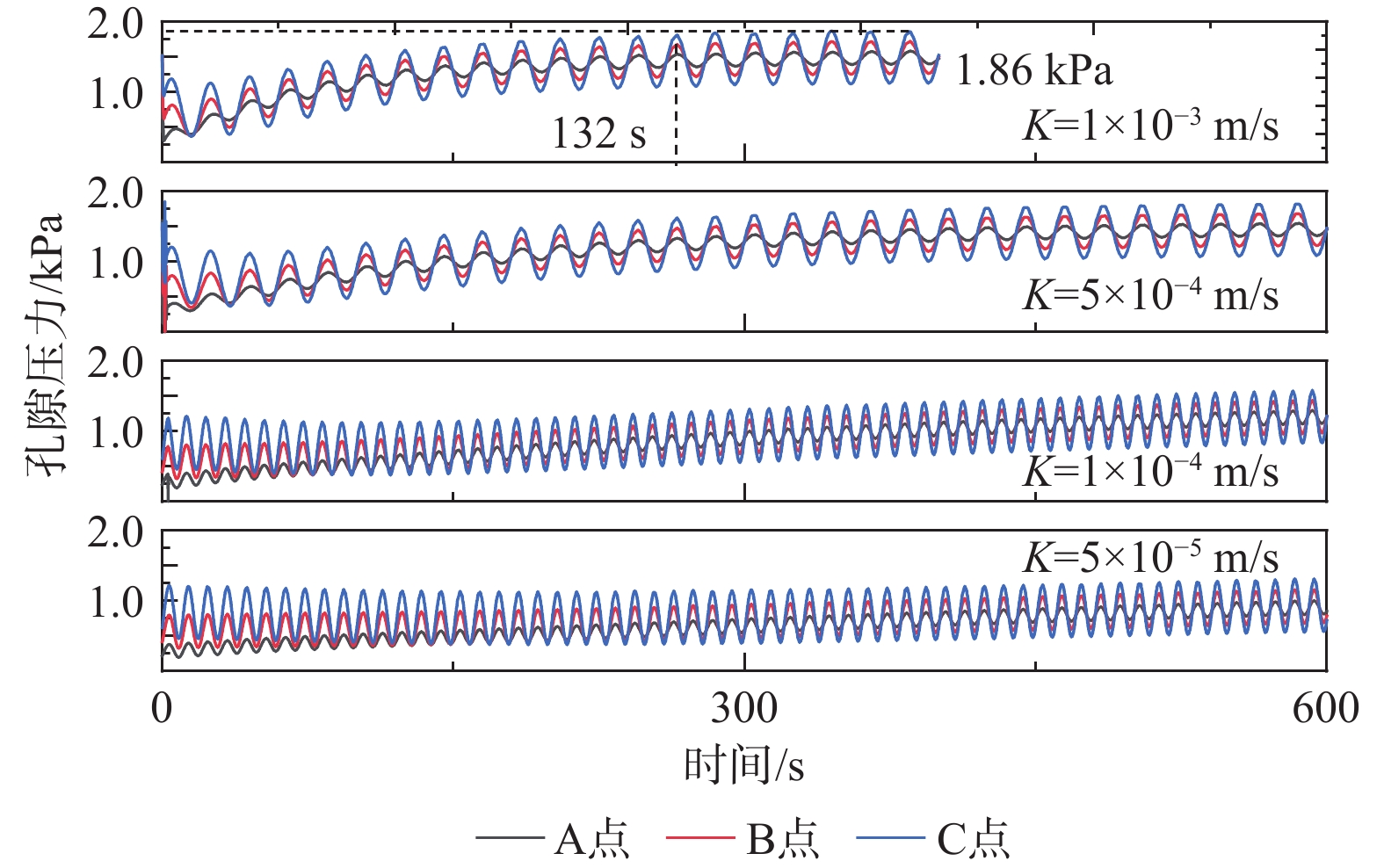
经典的地下水渗流理论是基于水均衡原理建立的,无法解释天然地震活动等外部荷载引起的井水位变化现象,也不利于深入认识地下水渗流运动在各种环境地质灾害中的作用。针对这一问题,基于流固耦合的动力学理论构建了地震波应力驱动承压含水层孔隙压力变化的数学模型,借助软件Comsol实现了对模型的有限元数值求解及影响因素分析,利用Cooper理论反演出井水位的变化特征,并将结果与强震引起川滇地区井水位的变化特征进行对比。结果表明:当地震波荷载作用于承压含水层时,孔隙压力会表现出与地震波同周期的振荡变化特征,且地震波振幅、频率以及水力坡度对孔隙压力的变化特征影响显著。地震波荷载施加初期,孔隙压力迅速增大,随后含水层中的部分水被缓慢排出,荷载压力逐渐转移到颗粒骨架上,孔隙压力的变化速率随之变缓并趋于新的平衡。井水位变化特征与孔隙压力密切相关,其振荡周期与变化形态与孔隙压力一致,但幅度不同,总体上表现出振荡上升且趋于稳定的变化特征,与川滇地区观测到的井水位变化形态基本相同。该成果对于应力作用下地下水渗流运动理论的建立和完善具有宝贵的探索意义,可以丰富和拓展传统地下水动力学和经典流固耦合理论的研究思路和应用领域。
Abstract:The classical groundwater seepage theory was established on the basis of the principle of water equilibrium, which cannot explain the phenomenon of well water level change caused by natural seismic activities and other external loads, and is not conducive to the in-depth understanding of the role of groundwater seepage movement in various environmental geological disasters. To solve this problem, a mathematical model of pore pressure change of a confined aquifer driven by seismic wave stress is constructed based on the fluid-structure coupling dynamic theory. The numerical verification of the model is realized by using the software Comsol. The change characteristics of well water level are inversely performed by using the Cooper theory, and the results are compared with the change characteristics of well water level caused by strong earthquakes in the Sichian-Yunnan region. The influencing factors of seepage movement of a confined aquifer under earthquake are studied by changing the simulation parameters. The results show that when seismic wave loads act on the confined aquifers, pore pressure oscillates in the same period as the seismic waves, and the amplitude, frequency and hydraulic slope of the seismic waves have significant effects on the pore pressure, while the coefficient of permeability and porosity have little effect on the change characteristics. At the initial stage of seismic wave loading, pore pressure increases rapidly, and then part of the water in the aquifer is slowly discharged. The load pressure gradually transfers to the granular framework, and the rate of change of pore pressure slows down and tends to reach a new equilibrium. The variation characteristics of well water level are closely related to pore pressure, and the oscillation period and variation pattern are consistent with pore pressure, but the amplitude is different. Generally, the oscillation rises and tends to be stable, which is basically the same with the variation pattern of well water level observed in the Sichuan-Yunnan region. The results are of valuable exploration significance for the establishment and improvement of groundwater seepage theory under stress, and can enrich and expand the research ideas and application fields of traditional groundwater dynamics and classical fluid-structure coupling theory.
-
Key words:
- seepage /
- pore pressure /
- well water level /
- fluid-structure coupling /
- theory of dynamics /
- numerical simulation
-

-
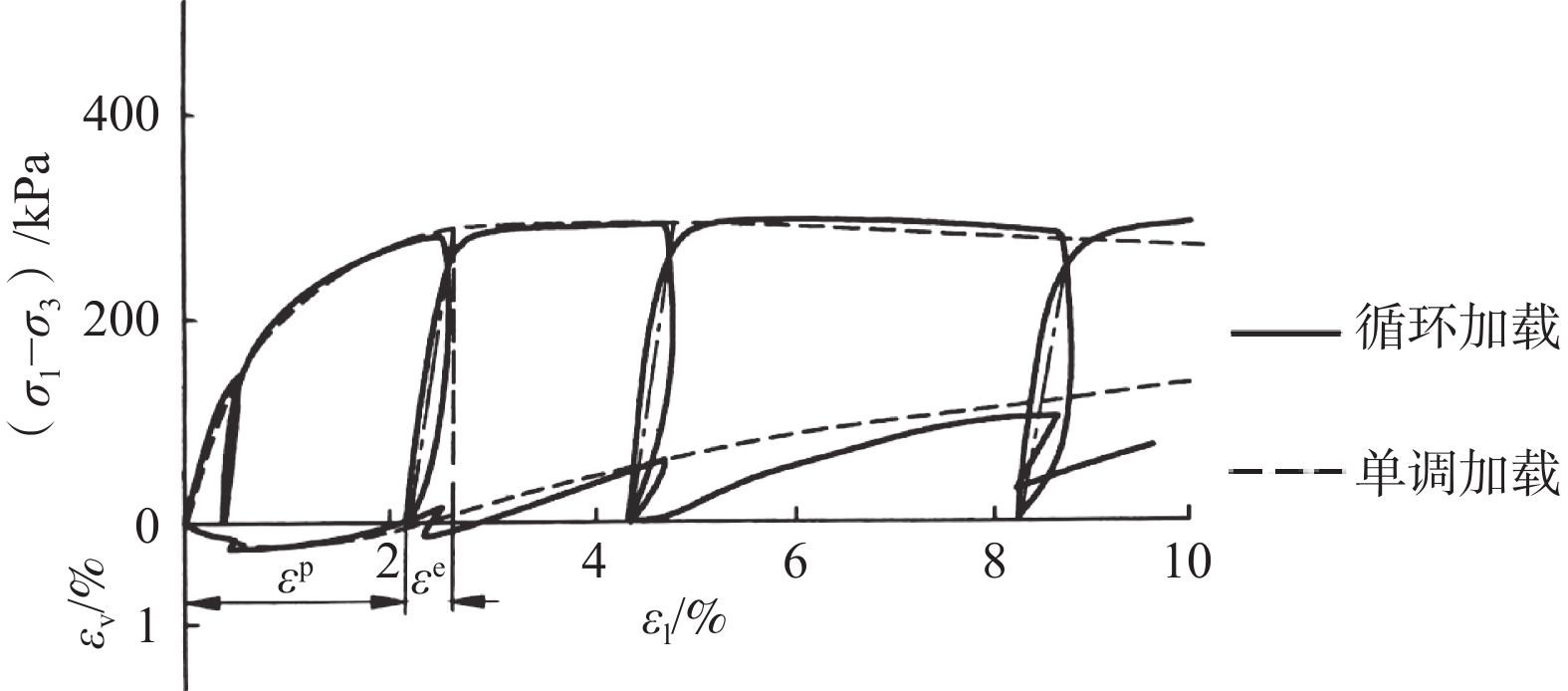
图 7 单调加载与循环加载三轴试验应变-应力变化对比曲线[27]
Figure 7.
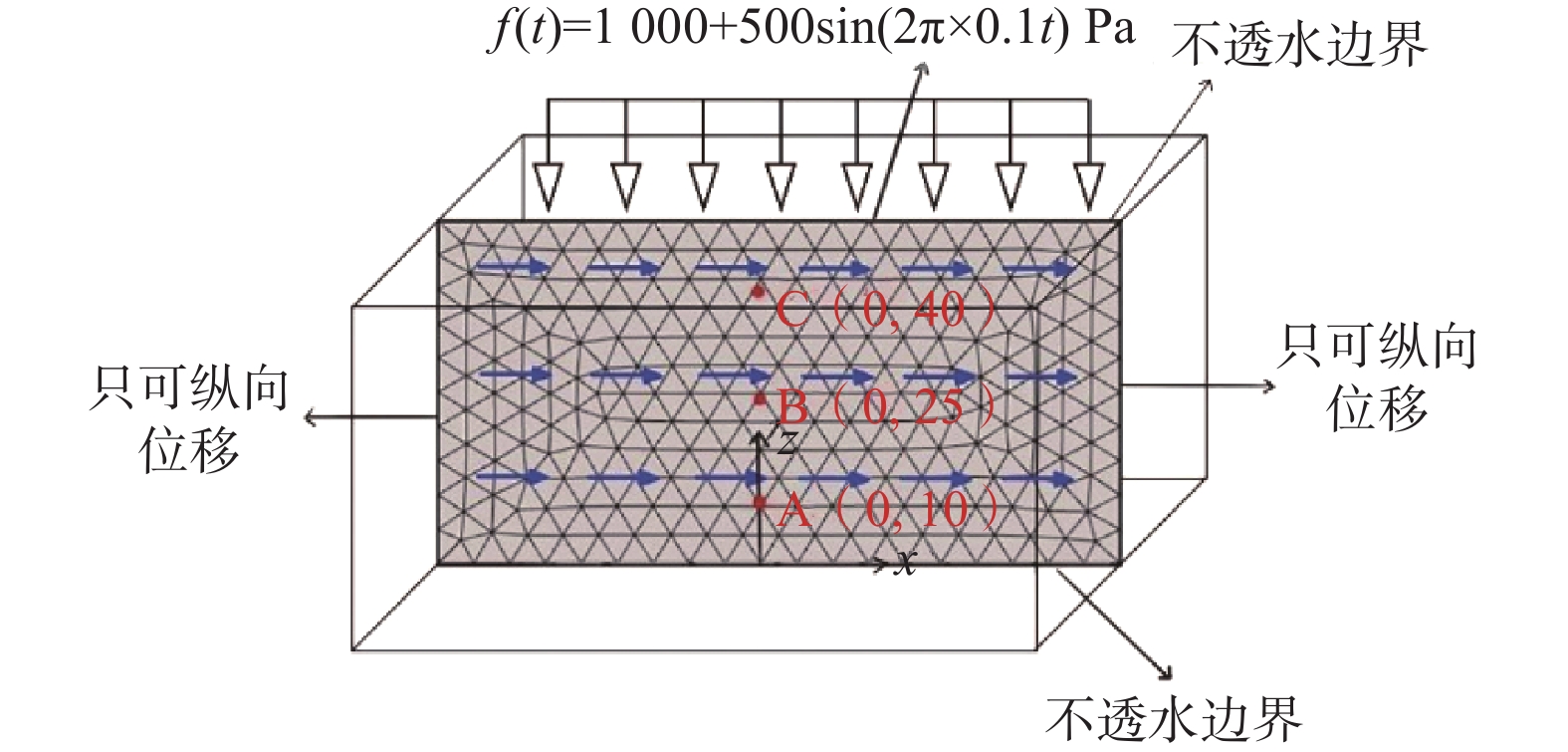
表 1 二维算例的含水层参数
Table 1. Aquifer model parameters for a 2D example
参数 数值 剪切模量/MPa 65 渗透系数/(m∙s−1) 0.001 液体密度/(kg∙m−3) 1000 泊松比 0.3 孔隙度 0.3 Biot系数 1 固体密度/(kg∙m−3) 2650 重力加速度/(m∙s−2) 9.8 -
[1] 王金生,王澎,刘文臣,等. 划分地下水源地保护区的数值模拟方法[J]. 水文地质工程地质,2004,31(4):83 − 86. [WANG Jinsheng,WANG Peng,LIU Wenchen,et al. Numerical simulation method for delineating protection zones of groundwater wellfields[J]. Hydrogeology & Engineering Geology,2004,31(4):83 − 86. (in Chinese with English abstract) doi: 10.3969/j.issn.1000-3665.2004.04.016
WANG Jinsheng, WANG Peng, LIU Wenchen, et al. Numerical simulation method for delineating protection zones of groundwater wellfields[J]. Hydrogeology and Engineering Geology, 2004, 31(4): 83-86. (in Chinese with English abstract) doi: 10.3969/j.issn.1000-3665.2004.04.016
[2] 贺国平,周东,杨忠山,等. 北京市平原区地下水资源开采现状及评价[J]. 水文地质工程地质,2005,32(2):45 − 48. [HE Guoping,ZHOU Dong,YANG Zhongshan,et al. Current status and evaluation of groundwater resources exploitation in the plain area of Beijing[J]. Hydrogeology & Engineering Geology,2005,32(2):45 − 48. (in Chinese with English abstract)
HE Guoping, ZHOU Dong, YANG Zhongshan, et al. Current status and evaluation of groundwater resources exploitation in the plain area of Beijing[J]. Hydrogeology & Engineering Geology, 2005, 32(2): 45-48. (in Chinese with English abstract)
[3] 刘鑫,左锐,王金生,等. 地下水位波动带三氮迁移转化过程研究进展[J]. 水文地质工程地质,2021,48(2):27 − 36. [LIU Xin,ZUO Rui,WANG Jinsheng,et al. Advances in researches on ammonia,nitrite and nitrate on migration and transformation in the groundwater level fluctuation zone[J]. Hydrogeology & Engineering Geology,2021,48(2):27 − 36. (in Chinese with English abstract)
LIU Xin, ZUO Rui, WANG Jinsheng, et al. Advances in researches on ammonia, nitrite and nitrate on migration and transformation in the groundwater level fluctuation zone[J]. Hydrogeology & Engineering Geology, 2021, 48(2): 27-36. (in Chinese with English abstract)
[4] 于勇,翟远征,郭永丽,等. 基于不确定性的地下水污染风险评价研究进展[J]. 水文地质工程地质,2013,40(1):115 − 123. [YU Yong,ZHAI Yuanzheng,GUO Yongli,et al. Risk assessment of groundwater pollution based on uncertainty[J]. Hydrogeology & Engineering Geology,2013,40(1):115 − 123. (in Chinese with English abstract)
YU Yong, ZHAI Yuanzheng, GUO Yongli, et al. Risk assessment of groundwater pollution based on uncertainty[J]. Hydrogeology & Engineering Geology, 2013, 40(1): 115-123. (in Chinese with English abstract)
[5] TRUESDELL C, TOUPIN R. Handbuch der Physik: The classical field theories-constitutive equations [M]. Berlin: Springer, 1960.
[6] TRUESDELL C. Sulle basi della termomeccanica[J]. Rend Lincei, 1957, 22(8): 33 − 38.
[7] ZIENKIEWICZ O C,SHIOMI T. Dynamic behaviour of saturated porous media:The generalized Biot formulation and its numerical solution[J]. International Journal for Numerical and Analytical Methods in Geomechanics,1984,8(1):71 − 96. doi: 10.1002/nag.1610080106
[8] ZIENKIEWICZ O C,CHANG C T,BETTESS P. Drained,undrained,consolidating and dynamic behaviour assumptions in soils[J]. Géotechnique,1980,30(4):385 − 395.
[9] ZIENKIEWICZ O C, LEUNG K H, HINTON E, et al. Liquefaction and permanent deformation under dynamic conditions: Numerical solution and constitutive relations[J]. Soil Mechanics-Transient and Cyclic Loads: Constitutive Relations and Numerical Treatment, 1982: 71 − 104.
[10] SIMON B R,ZIENKIEWICZ O C,PAUL D K. An analytical solution for the transient response of saturated porous elastic solids[J]. International Journal for Numerical and Analytical Methods in Geomechanics,1984,8(4):381 − 398. doi: 10.1002/nag.1610080406
[11] HUANG Maosong,YUE Zhongqi,THAM L G,et al. On the stable finite element procedures for dynamic problems of saturated porous media[J]. International Journal for Numerical Methods in Engineering,2004,61(9):1421 − 1450. doi: 10.1002/nme.1115
[12] 赵成刚,王进廷,史培新,等. 流体饱和两相多孔介质动力反应分析的显式有限元法[J]. 岩土工程学报,2001,23(2):178 − 182. [ZHAO Chenggang,WANG Jinting,SHI Peixin,et al. Dynamic analysis of fluid-saturated porous media by using explicit finite element method[J]. Chinese Journal of Geotechnical Engineering,2001,23(2):178 − 182. (in Chinese with English abstract)
ZHAO Chenggang, WANG Jinting, SHI Peixin, et al. Dynamic analysis of fluid-saturated porous media by using explicit finite element method[J]. Chinese Journal of Geotechnical Engineering, 2001, 23(2): 178-182. (in Chinese with English abstract)
[13] 刘宝,苏谦,刘亭,等. 饱和多孔介质不同动力耦合形式数值分析[J]. 振动与冲击,2017,36(9):146 − 152. [LIU Bao,SU Qian,LIU Ting,et al. Numerical simulation for different dynamic coupling forms of saturated porous media[J]. Journal of Vibration and Shock,2017,36(9):146 − 152. (in Chinese with English abstract)
LIU Bao, SU Qian, LIU Ting, et al. Numerical simulation for different dynamic coupling forms of saturated porous media[J]. Journal of Vibration and Shock, 2017, 36(9): 146-152. (in Chinese with English abstract)
[14] 杨贝贝,李伟华,赵成刚,等. 基于Comsol的饱和多孔介质动力方程的数值模拟及应用[J]. 地震工程学报,2017,39(2):321 − 328. [YANG Beibei,LI Weihua,ZHAO Chenggang,et al. Numerical simulation and application of dynamic equations of saturated porous media based on COMSOL[J]. China Earthquake Engineering Journal,2017,39(2):321 − 328. (in Chinese with English abstract)
YANG Beibei, LI Weihua, ZHAO Chenggang, et al. Numerical simulation and application of dynamic equations of saturated porous media based on COMSOL[J]. China Earthquake Engineering Journal, 2017, 39(2): 321-328. (in Chinese with English abstract)
[15] 薛禹群. 地下水动力学[M]. 2版. 北京: 地质出版社, 1997: 1 − 10
XUE Yuqun. Groundwater hydraulics[M]. 2nd ed. Beijing: Geological Publishing House, 1997: 1 − 10. (in Chinese)
[16] COOPER H H Jr,BREDEHOEFT J D,PAPADOPULOS I S,et al. The response of well-aquifer systems to seismic waves[J]. Journal of Geophysical Research,1965,70(16):3915 − 3926. doi: 10.1029/JZ070i016p03915
[17] ROELOFFS E,QUILTY E,SCHOLTZ C H. Case 21 water level and strain changes preceding and following the August 4,1985 Kettleman Hills,California,earthquake[J]. Pure and Applied Geophysics,1997,149(1):21 − 60. doi: 10.1007/BF00945160
[18] DOAN M L, BRODSKY E E. Tidal analysis of water level in continental boreholes A tutorial Version 2.2[D]. University of California, 2006.
[19] HSIEH P A,BREDEHOEFT J D,FARR J M. Determination of aquifer transmissivity from earth tide analysis[J]. Water Resources Research,1987,23(10):1824 − 1832. doi: 10.1029/WR023i010p01824
[20] WANG Chiyuen. Tidal response of groundwater in a leaky aquifer application to Oklahoma[J]. Water Resources Research,2018,54(10):8019 − 8033. doi: 10.1029/2018WR022793
[21] ZHU Aiyu,WANG Chiyuen. Response of leaky aquifers to earth tides:Interpreted with numerical simulation[J]. Journal of Hydrology,2020,581:124458. doi: 10.1016/j.jhydrol.2019.124458
[22] 刘洋. 土动力学基本原理[M]. 北京: 清华大学出版社, 2019
LIU Yang. Fundamentals of soil dynamics[M]. Beijing: Tsinghua University Press, 2019. (in Chinese)
[23] XU Chengshun,FENG Chaoqun,SONG Jia,et al. A novel completely explicit algorithm to solve dynamic u-p equations of saturated soil[J]. Soil Dynamics and Earthquake Engineering,2021,141:106452. doi: 10.1016/j.soildyn.2020.106452
[24] HUANG Maosong,WU Shiming,ZIENKIEWICZ O C. Incompressible or nearly incompressible soil dynamic behaviour:A new staggered algorithm to circumvent restrictions of mixed formulation[J]. Soil Dynamics and Earthquake Engineering,2001,21(2):169 − 179. doi: 10.1016/S0267-7261(00)00105-6
[25] ZIENKIEWICZ O, LEUNG K, HINTON E, et al. Liquefaction and permanent deformation under dynamic conditions: Numerical solution and constitutive relations[J]. Soil Mechanics-Transient and Cyclic Loads: Constitutive Relations and Numerical Treatment, 1982: 71 − 104.
[26] 重庆市地震局. 重庆市地震监测志[M]. 北京: 地震出版社, 2011.
Chongqing Earthquake Ageny. Chongqing earthquake monitoring records[M]. Beijing: Seismological Press,2011. (in Chinese)
[27] XING Yixuan,LIU Quan,HU Rui, et al. A general numerical model for water level response to harmonic disturbances in aquifers considering wellbore effects[J]. Journal of Hydrology,2022,609:127678. doi: 10.1016/j.jhydrol.2022.127678
[28] KONDNER R L. Hyperbolic stress-strain response:Cohesive soils[J]. Journal of the Soil Mechanics and Foundations Division,1963,89(1):115 − 143. doi: 10.1061/JSFEAQ.0000479
[29] LIN C H,LEE V W,TRIFUNAC M D. The reflection of plane waves in a poroelastic half-space saturated with inviscid fluid[J]. Soil Dynamics and Earthquake Engineering,2005,25(3):205 − 223. doi: 10.1016/j.soildyn.2004.10.009
-



 下载:
下载: