考虑土拱效应及中主应力影响的桩间挡板土压力计算方法

尤静霖; 王士杰; 王昊岚

doi:10.16030/j.cnki.issn.1000-3665.202201032

考虑土拱效应及中主应力影响的桩间挡板土压力计算方法

河北农业大学城乡建设学院, 河北保定　071001

基金项目: 河北省建设科技研究指导性计划项目(2016-236)

详细信息

作者简介: 尤静霖（1997-），女，硕士研究生，主要从事边坡稳定性分析与滑坡防治研究。E-mail：Yoojiln@163.com

通讯作者: 王士杰（1963-），男，博士，教授，博士研究生导师，主要从事岩土工程教学与科研工作。E-mail：wshj_wshj@163.com

中图分类号: TU432

收稿日期: 2022-01-17

修回日期: 2022-03-23

刊出日期: 2022-09-15

A calculation method of earth pressure on sheeting between two piles considering soil arching effect and intermediate principal stress

Urban and Rural Construction Institute, Hebei Agricultural University, Baoding, Hebei　071001, China

More Information

Corresponding author: WANG Shijie, wshj_wshj@163.com

Received Date: 17 January 2022

Revised Date: 23 March 2022

Publish Date: 15 September 2022

摘要

摘要:
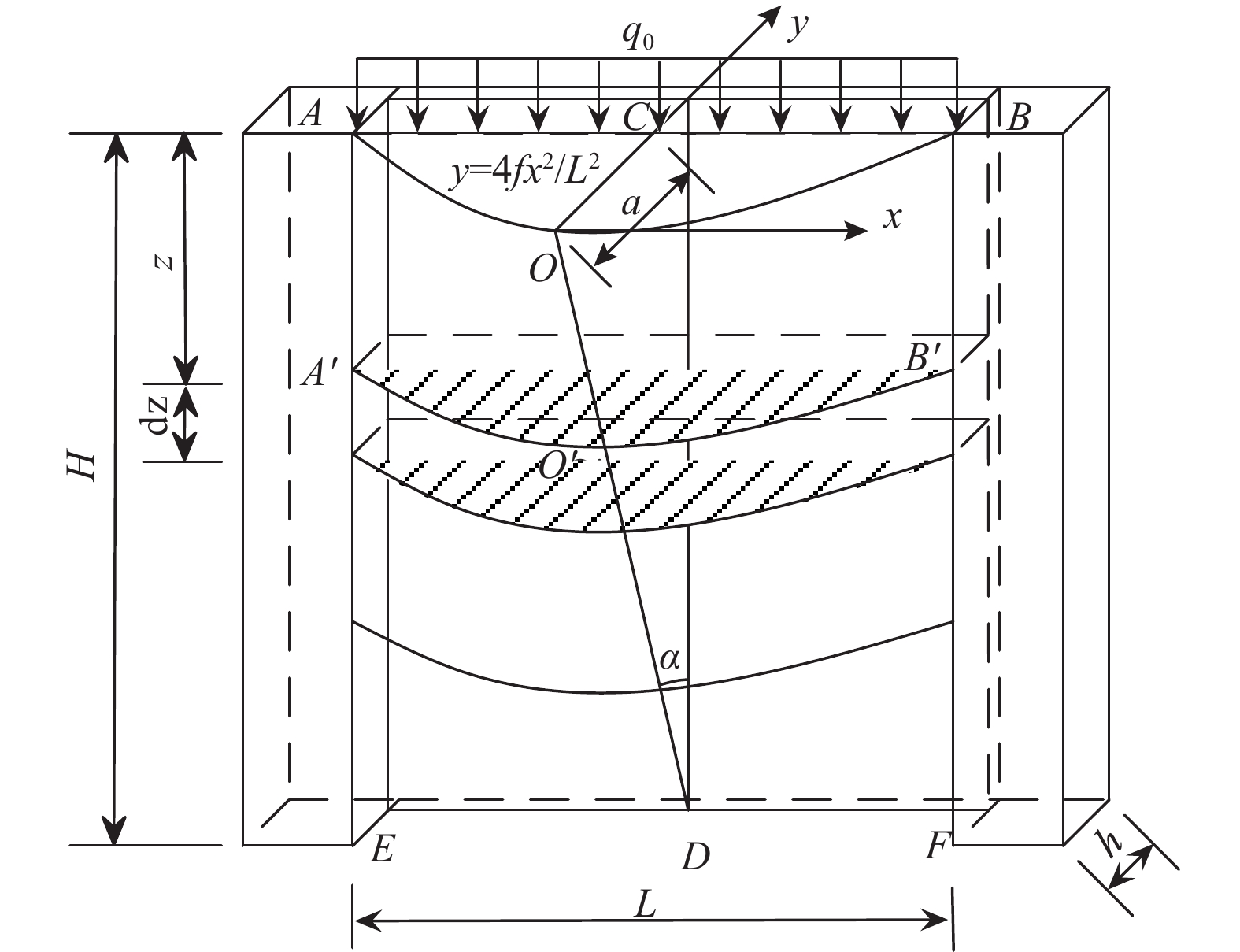
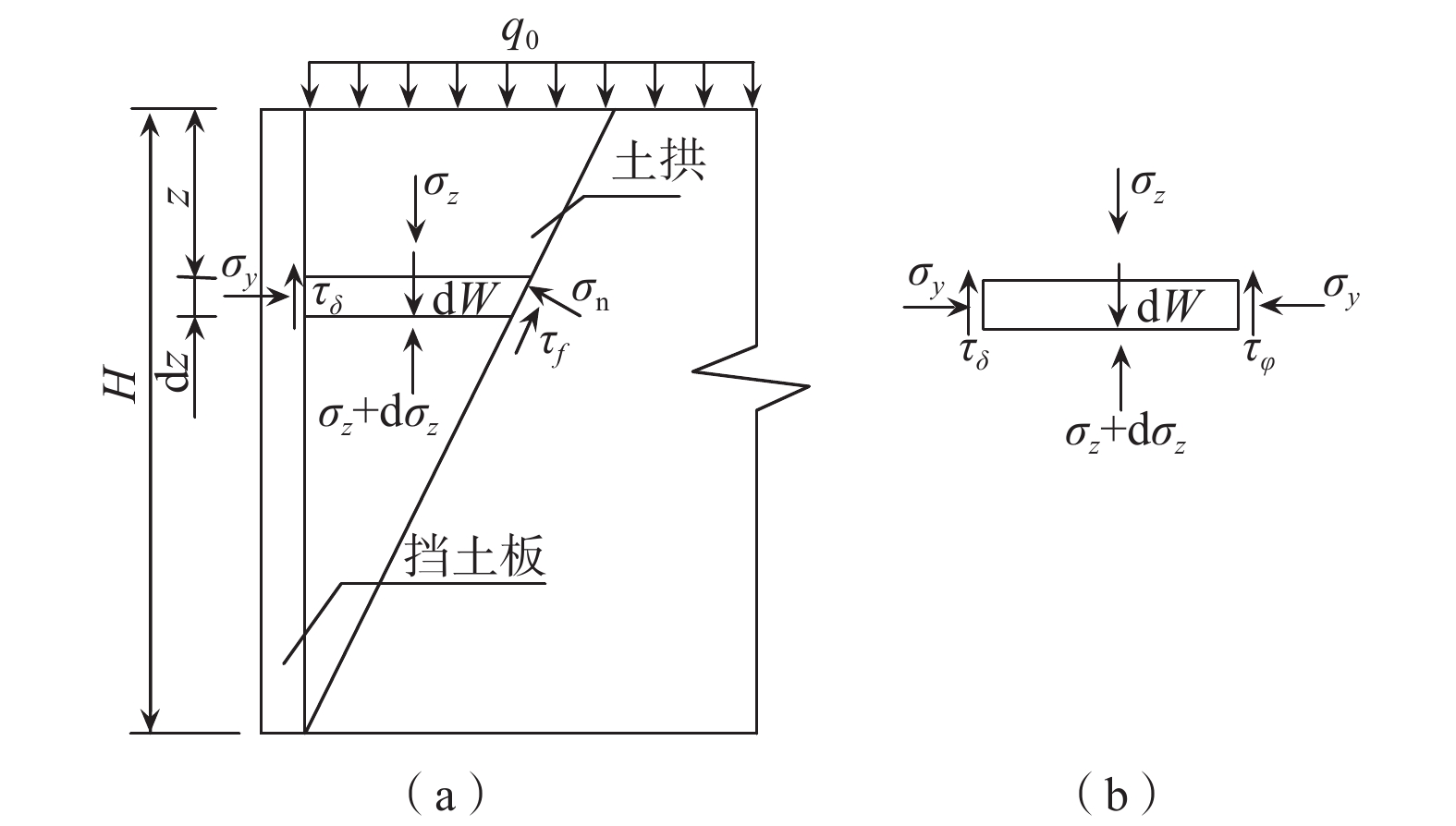
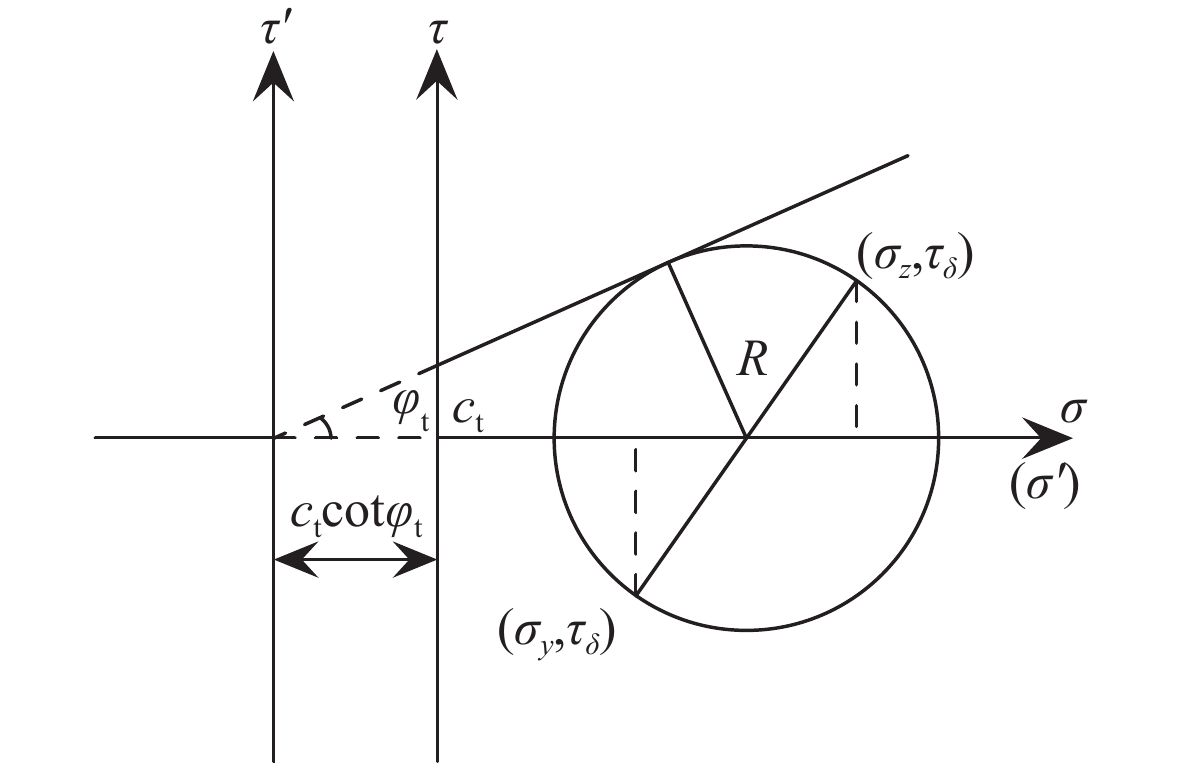
基于M-C强度准则的经典土压力理论，因未考虑土拱效应及中主应力的影响，导致土压力理论计算值较实际值明显偏大。在该方面，现有研究成果亦不能同时考虑二者的影响。为考虑土拱效应、中主应力和土体黏聚力等对桩间挡板土压力的综合影响，借助统一强度理论，对桩间土拱强度进行分析，给出了桩间挡板土压力计算的新方法。另外，基于Lode参数与应变类型的关系，提出了统一强度指标${\varphi _{\text{t}}}$的实用计算方法。结合工程实例，将计算结果与已有相关研究成果和现行规范确定的土压力进行了对比分析。结果表明，采用该方法，不同中主应力系数b值条件下，计算所得总主动土压力约为经典朗肯理论土压力的1/3~1/2，与实测土压力分布规律基本吻合。研究成果对桩板支挡结构的优化设计、降低工程造价，具有重要理论指导意义和工程实用价值。
- 桩板墙 /
- 挡板 /
- 土压力 /
- 土拱效应 /
- 中主应力 /
- 统一强度理论
Abstract:
The classical theories of earth pressure are based on the M-C strength criterion, which do not consider the influence of soil arching effect and intermediate principal stress on earth pressure, so the theoretical earth pressures are significantly larger than the actual values. In this respect, the existing research results also cannot consider the impact of both. In order to consider the comprehensive effect of soil arching effect, intermediate principal stress and soil cohesion on the earth pressure on the sheeting between two piles, the unified strength theory is used to analyze the strength of soil arching between piles, and a new method for calculating the earth pressure on the sheeting between piles is derived. In addition, based on the relationship between the Lode parameters and strain types, a practical calculation method of the unified strength index ${\varphi _{\text{t}}}$ is proposed. Finally, combined with an engineering example, the calculated results are compared with the existing research results and the earth pressure determined by using the current code. The results indicate that the total active earth pressure calculated with this method is about 1/3~1/2 of that calculated by the traditional Rankine theory under the condition of different b values, and it is in accordance with the distribution law of the measured earth pressure. The research results are of important theoretical significance and practical value for the optimal design of pile-sheet retaining and the reduction of engineering cost.
- pile-sheet retaining /
- sheeting /
- earth pressure /
- soil arching effect /
- intermediate principal stress /
- unified strength theory

HTML全文

图 1 桩板墙土拱效应示意图

Figure 1.

下载: 全尺寸图片幻灯片

图 2 土拱受力简图

Figure 2.

下载: 全尺寸图片幻灯片

图 3 板后土拱随深度变化示意图

Figure 3.

下载: 全尺寸图片幻灯片

图 4 板后土拱受力分析示意图

Figure 4.

下载: 全尺寸图片幻灯片

图 5 统一强度理论的极限应力圆

Figure 5.

下载: 全尺寸图片幻灯片

图 6 挡板土压力值随深度变化曲线

Figure 6.

下载: 全尺寸图片幻灯片

表 1 土层主要物理力学参数

Table 1. Main physical and mechanical parameters of different soil layers

序号	土层名称	土层厚度/m	重度/ （kN·m⁻³）	黏聚力/ kPa	内摩擦角/（°）
①	杂填土	3.26	18.5	6.0	13.0
②	含碎石粉质黏土	5.50	19.5	22.0	17.0
③₁	松散碎石	2.00	20.0	8.0	22.0
③₂	稍密碎石	4.00	20.5	5.0	25.0
④₁	稍密卵石	4.50	21.0	−	28.0
④₂	中密卵石	0.74	21.5	−	32.0

下载: 导出CSV

表 2 不同方法计算所得挡板总主动土压力

Table 2. Total active earth pressure of baffle calculated with different methods

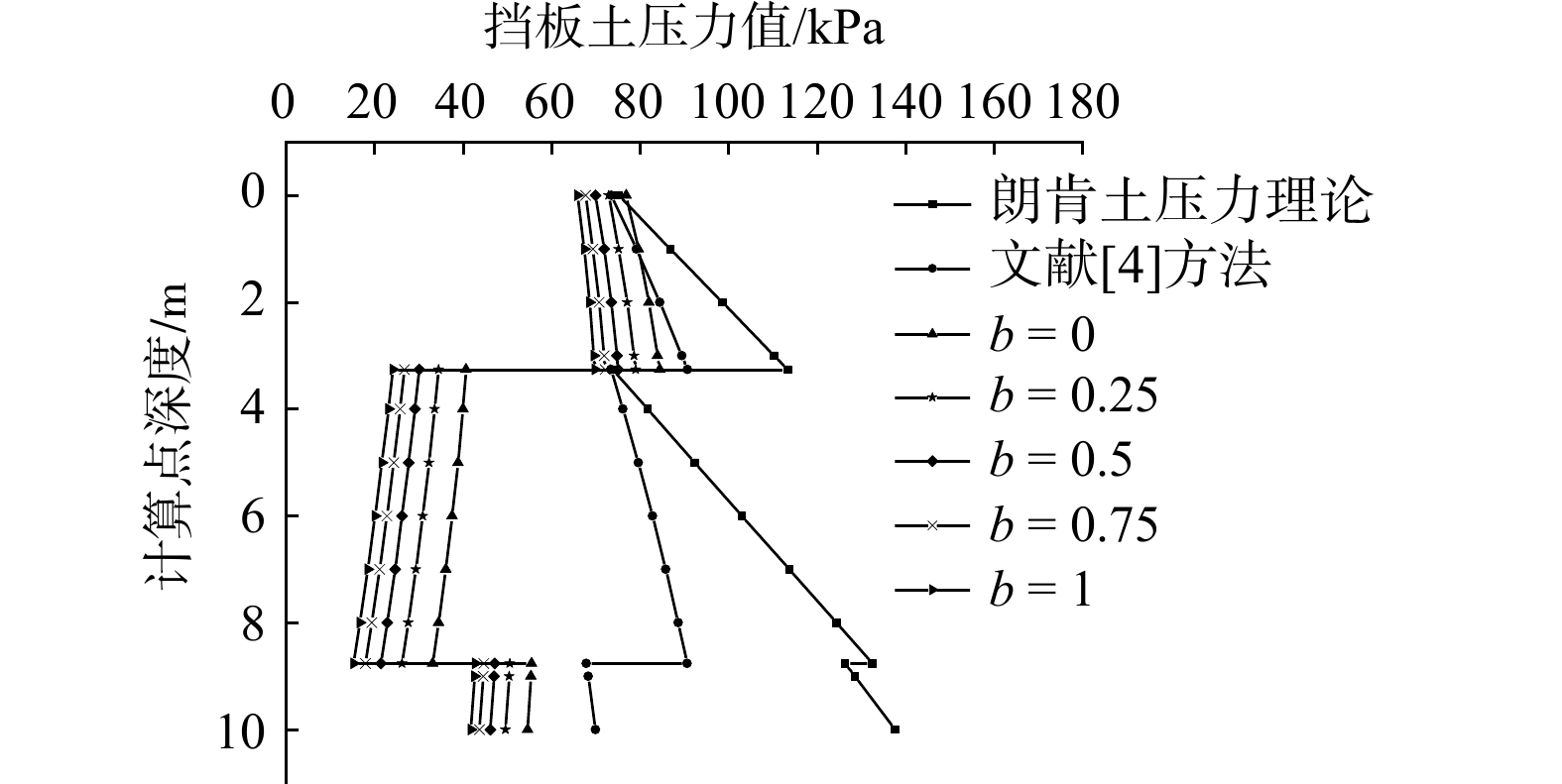
计算方法	总主动土压力/（kN·m⁻¹）
朗肯理论	1038.46
文献[4]方法	808.37
本文方法（b = 0）	537.62
本文方法（b = 0.25）	479.69
本文方法（b = 0.5）	438.62
本文方法(b = 0.75）	407.97
本文方法（b = 1.0）	384.19

下载: 导出CSV

参考文献(17)

[1]	向俐蓉,陈伟志,郭在旭,等. 大型滑坡抗滑桩-桩板结构受力变形研究[J]. 水文地质工程地质,2021,48(2):125 − 131. [XIANG Lirong,CHEN Weizhi,GUO Zaixu,et al. A study of the deformation of anti-slide pile and pile-plate structure in large landslide[J]. Hydrogeology & Engineering Geology,2021,48(2):125 − 131. (in Chinese with English abstract) doi: 10.16030/j.cnki.issn.1000-3665.201911032
[2]	王佳宇,曹文贵,王雨波,等. 基于圆弧主应力迹线的黏性土主动土压力分析[J]. 水文地质工程地质,2021,48(6):81 − 88. [WANG Jiayu,CAO Wengui,WANG Yubo,et al. An analysis of active earth pressure of cohesive soil based on the layering of principal stress traces[J]. Hydrogeology & Engineering Geology,2021,48(6):81 − 88. (in Chinese with English abstract) doi: 10.16030/j.cnki.issn.1000-3665.202103006
[3]	张健, 胡瑞林, 刘海斌, 等. 基于统一强度理论朗肯土压力的计算研究[J]. 岩石力学与工程学报, 2010, 29（增刊1）: 3169 − 3176 ZHANG Jian, HU Ruilin, LIU Haibin, et al. Calculation study of Rankine earth pressure based on unified strength theory[J]. Chinese Journal of Rock Mechanics and Engineering, 2010, 29（Sup 1）: 3169 − 3176. （in Chinese with English abstract）
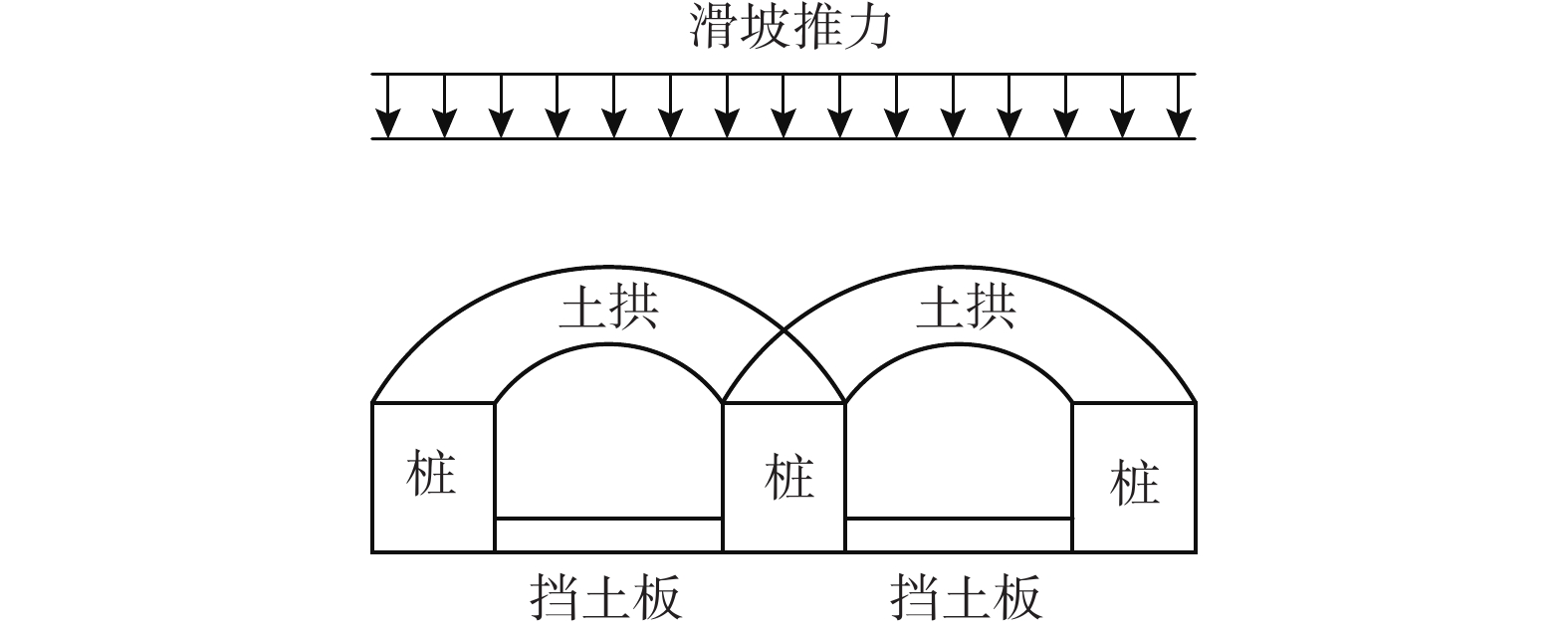
[4]	刘力生, 吴曙光. 基于土拱效应的悬臂式抗滑桩桩间挡板土压力计算[J]. 岩土工程学报, 2015, 37（增刊2）: 153 − 157. LIU Lisheng, WU Shuguang. Calculation of earth pressure on baffle of cantilever anti-slide piles based on soil arching effects[J]. Chinese Journal of Geotechnical Engineering, 2015, 37（Sup 2）: 153 − 157. （in Chinese with English abstract）
[5]	范文,沈珠江,俞茂宏. 基于统一强度理论的土压力极限上限分析[J]. 岩土工程学报,2005,27(10):1147 − 1153. [FAN Wen,SHEN Zhujiang,YU Maohong. Upper-bound limit analysis of earth pressure based on unified strength theory[J]. Chinese Journal of Geotechnical Engineering,2005,27(10):1147 − 1153. (in Chinese with English abstract) doi: 10.3321/j.issn:1000-4548.2005.10.007
[6]	应捷, 廖红建, 蒲武川. 平面应变状态下基于统一强度理论的土压力计算[J]. 岩石力学与工程学报, 2004, 23（增刊1）: 4315 − 4318 YING Jie, LIAO Hongjian, PU Wuchuan. Earth pressure theory based on unified strength theory under plane strain state[J]. Chinese Journal of Rock Mechanics and Engineering, 2004, 23（Sup 1）: 4315 − 4318. （in Chinese with English abstract）
[7]	张建云. 基于土拱效应的桩板墙与岩土体相互作用及其优化设计研究[D]. 西安: 长安大学, 2017 ZHANG Jianyun. The interaction between sheet-pile wall and rock-soil body and its optimization research based on the soil arching effect[D]. Xi’an: Chang’an University, 2017. （in Chinese with English abstract）
[8]	胡焕校,刘静,吴海涛,等. 抗滑桩桩后土拱效应特征的三维数值研究[J]. 工业建筑,2010,40(3):88 − 93. [HU Huanxiao,LIU Jing,WU Haitao,et al. Three-dimensional numerical analysis of soil arching effect surrounding anti-slide pile[J]. Industrial Construction,2010,40(3):88 − 93. (in Chinese with English abstract) doi: 10.13204/j.gyjz2010.03.016
[9]	陈强,董桂城,王超,等. 基于透明土技术的桩后土拱效应特征分析[J]. 西南交通大学学报,2020,55(3):509 − 522. [CHEN Qiang,DONG Guicheng,WANG Chao,et al. Characteristics analysis of soil arching effect behind pile based on transparent soil technology[J]. Journal of Southwest Jiaotong University,2020,55(3):509 − 522. (in Chinese with English abstract) doi: 10.3969/j.issn.0258-2724.20190744
[10]	李婷,肖世国. 考虑土拱效应的抗滑桩桩间挡土板压力计算方法[J]. 中国地质灾害与防治学报,2019,30(4):85 − 91. [LI Ting,XIAO Shiguo. Calculation method of earth pressure on retaining plates between adjacent stabilizing piles considering potential soil arching effect[J]. The Chinese Journal of Geological Hazard and Control,2019,30(4):85 − 91. (in Chinese with English abstract) doi: 10.16031/j.cnki.issn.1003-8035.2019.04.12
[11]	刘杰,吴昭云,路利民,等. 考虑三维土拱效应的桩间挡板土压力计算[J]. 中外公路,2020,40(5):17 − 21. [LIU Jie,WU Zhaoyun,LU Limin,et al. Calculation of earth pressure on baffle between two piles considering three-dimensional soil arching effect[J]. Journal of China & Foreign Highway,2020,40(5):17 − 21. (in Chinese) doi: 10.14048/j.issn.1671-2579.2020.05.005
[12]	王仲仁. Lode参数的物理实质及其对塑性流动的影响[J]. 固体力学学报,2006,27(3):277 − 282. [WANG Zhongren. Physical essence of lode parameter and its effect on plastic flow[J]. Acta MechanicaSolidaSinica,2006,27(3):277 − 282. (in Chinese with English abstract) doi: 10.3969/j.issn.0254-7805.2006.03.010
[13]	国家铁路局. 铁路路基支挡结构设计规范: TB 10025—2019[S]. 北京: 中国铁道出版社, 2019 National Railway Administration of the People’s Republic of China. Code for design of retaining structures of railway earthworks: TB 10025—2019[S]. Beijing: China Railway Publishing House, 2019. （in Chinese）
[14]	重庆市建设委员会, 重庆市国土资源和房屋管理局. 地质灾害防治工程设计规范: DB 50/5029—2004[S]. 重庆: 重庆市建设委员会, 2004 Construction Commission of Chongqing, Bureau of Land Resources and Housing Management of Chongqing Municipality. Design standard of geological disaster prevention and control: DB 50/5029—2004 [S]. Chongqing: Construction Commission of Chongqing, 2004. （in Chinese）
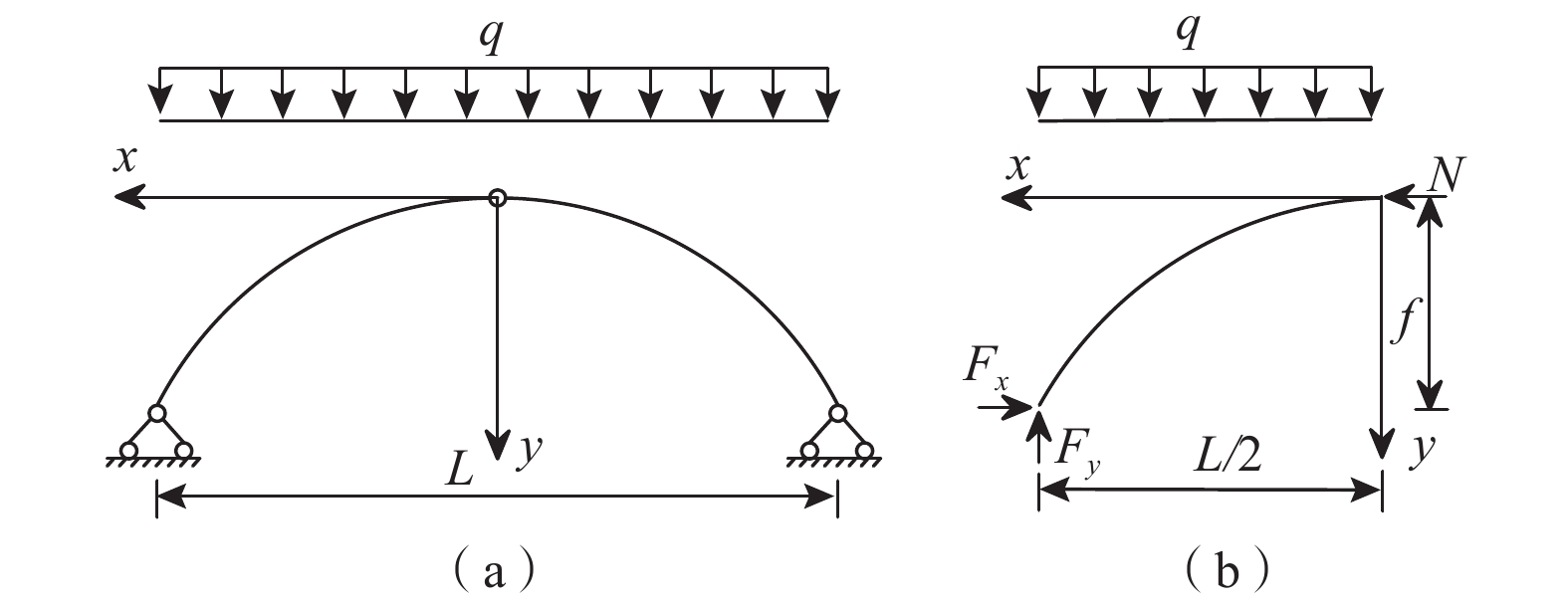
[15]	魏德芳,赵继德. 三铰拱的合理拱轴线方程[J]. 曲阜师范大学学报(自然科学版),1994,20(3):80 − 83. [WEI Defang,ZHAO Jide. On appropriate asis equation of tri-hinge Arches[J]. Journal of Qufu Normal University (Natural Science),1994,20(3):80 − 83. (in Chinese with English abstract)
[16]	焦赟,白千千. 基于土拱效应下的地震作用与抗滑桩桩间距关系分析[J]. 水文地质工程地质,2013,40(5):58 − 63. [JIAO Yun,BAI Qianqian. An analysis of the relationship between seismic action and pile spacing of anti-slide piles based on soil arch effect[J]. Hydrogeology & Engineering Geology,2013,40(5):58 − 63. (in Chinese with English abstract) doi: 10.16030/j.cnki.issn.1000-3665.2013.05.009
[17]	代军,胡岱文,吴曙光. 桩锚支挡结构体系挡板土压力试验研究[J]. 重庆建筑大学学报,2001,23(4):48 − 54. [DAI Jun,HU Daiwen,WU Shuguang. Experimental study of soil stress on the holding sheet of pile-anchor holding-block structure system[J]. Journal of Chongqing Jianzhu University,2001,23(4):48 − 54. (in Chinese with English abstract)